Algebra

Algebra

Easy Questions
Moderate Questions
Difficult Questions

Aptitude

Simplification
Number System
Fractions
Elementary Algebra
LCM and HCF
Approximation
Unitary Method
Linear Equation
Quadratic Equation
Discount
Average
Surds and Indices
Percentage
Square root and cube root
Order of Magnitude
Work and Wages
Odd Man Out and Series
Algebra
Profit and Loss
Stocks and Shares
True Discount
Ratio, Proportion
Partnership
Alligation or Mixture
Time and Work
Pipes and Cistern
Speed, Time and Distance
Problem on Trains
Height and Distance
Banker's Discount
Boats and Streams
Races and games
Problems on Ages
Clocks and Calendars
Simple interest
Compound Interest
Sets and Functions
Area and Perimeter
Volume and Surface Area of Solid Figures
Sequences and Series
Plane Geometry
Logarithm
Probability
Permutation and Combination
Statistics
Mensuration
Trigonometry
Aptitude miscellaneous
  1. If 2x = √a +
    1
    		 , a > 0, then the value of
    x
    		 is
    √ax − √x2 − 1








  1. View Hint View Answer Discuss in Forum

    2x = √a +
    1
    √a

    On squaring both sides,
    4x2 = a +
    1
    		 + 2
    a

    ⇒  4x2 – 4 = a +
    1
    		 + 2 – 4
    a

    = a +
    1
    		 – 2
    a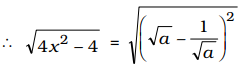


    = √a −
    1
    √a

    ∴  √x2 − 1 =
    1
    		√a −
    1
    2√a

    ∴  Expression =
    √x2 − 1
    x − √x2 − 1


    =
    1
    		 (a − 1)
    2

    Correct Option: C

    2x = √a +
    1
    √a

    On squaring both sides,
    4x2 = a +
    1
    		 + 2
    a

    ⇒  4x2 – 4 = a +
    1
    		 + 2 – 4
    a

    = a +
    1
    		 – 2
    a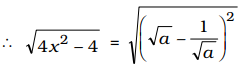


    = √a −
    1
    √a

    ∴  √x2 − 1 =
    1
    		√a −
    1
    2√a

    ∴  Expression =
    √x2 − 1
    x − √x2 − 1


    =
    1
    		 (a − 1)
    2

  1. If √ 1 +
    27
    		 = 1 +
    x
    		, then x equals
    16913








  1. View Hint View Answer Discuss in Forum


    ⇒ 
    14
    		 = 1 +
    x
    1313

    ⇒  1 +
    1
    		 = 1 +
    x
    1313

    ⇒  x = 1

    Correct Option: A


    ⇒ 
    14
    		 = 1 +
    x
    1313

    ⇒  1 +
    1
    		 = 1 +
    x
    1313

    ⇒  x = 1

  1. If  
    2p
    		 =
    1
    		, then the value of p +
    1
    		 is
    pr − 2p + 14p








  1. View Hint View Answer Discuss in Forum

    2p
    		 =
    1
    p2 − 2p + 14

    On dividing numerator and denominator by p, we get,
    2p
    		 =
    1
    p − 2 + (1/p)4

    ⇒  p +
    1
    		 – 2 = 8
    p

    ⇒  p +
    1
    		 = 8 + 2 = 10
    p

    Correct Option: D

    2p
    		 =
    1
    p2 − 2p + 14

    On dividing numerator and denominator by p, we get,
    2p
    		 =
    1
    p − 2 + (1/p)4

    ⇒  p +
    1
    		 – 2 = 8
    p

    ⇒  p +
    1
    		 = 8 + 2 = 10
    p

  1. If 2s = a + b + c, then the value of s(s – c) + (s – a) (s – b) is








  1. View Hint View Answer Discuss in Forum

    2s = a + b + c

    ∴  s (s – c) =
    a + b + c
    a + b + c
    		 − c
    22

    =
    (a + b + c)(a + b − c)
    4

    Again, (s – a) (s – b)
    =
    1
    		 (2s – 2a) (2s – 2b)
    4

    =
    1
    		 (a + b + c – 2a) (a + b + c – 2b)
    4

    =
    1
    		 (b + c – a) (a + c – b)
    4

    ∴  s (s – c) + (s – a) (s – b)
    =
    1
    		 [(a + b + c) (a + b – c) + (b + c – a) (a + c – b)]
    4

    =
    1
    		[(a + b)2 – c2 + ab + ac – a2 + bc + c2 – ac – b2 – bc + ab]
    4

    =
    1
    		(a2 + b2 + 2ab – c2 + ab + ac – a2 + bc + c2 – ac – b2 – bc + ab)
    4

    =
    1
    		 × 4ab = ab
    4

    Correct Option: A

    2s = a + b + c

    ∴  s (s – c) =
    a + b + c
    a + b + c
    		 − c
    22

    =
    (a + b + c)(a + b − c)
    4

    Again, (s – a) (s – b)
    =
    1
    		 (2s – 2a) (2s – 2b)
    4

    =
    1
    		 (a + b + c – 2a) (a + b + c – 2b)
    4

    =
    1
    		 (b + c – a) (a + c – b)
    4

    ∴  s (s – c) + (s – a) (s – b)
    =
    1
    		 [(a + b + c) (a + b – c) + (b + c – a) (a + c – b)]
    4

    =
    1
    		[(a + b)2 – c2 + ab + ac – a2 + bc + c2 – ac – b2 – bc + ab]
    4

    =
    1
    		(a2 + b2 + 2ab – c2 + ab + ac – a2 + bc + c2 – ac – b2 – bc + ab)
    4

    =
    1
    		 × 4ab = ab
    4

  1. If
    3 − 5x
    		 +
    3 − 5y
    		 +
    3 − 5z
    		 = 0, the value of
    2
    		 +
    2
    		 +
    2
    		 is
    2x2y2zxyz








  1. View Hint View Answer Discuss in Forum

    3 − 5x
    		 +
    3 − 5y
    		 +
    3 − 5z
    		 = 0
    2x2y2z

    ⇒ 
    3
    		−
    5x
    		 +
    3
    		−
    5y
    		 +
    3
    		 −
    5z
    		 = 0
    2x2x2y2y2z2z

    ⇒ 
    3
    		+
    3
    		+
    3
    		 −
    5
    		 −
    5
    		 −
    5
    		 = 0
    2x2y2z222

    ⇒ 
    3
    		+
    3
    		+
    3
    		 =
    3 × 5
    2x2y2z2

    ⇒ 
    1
    		 +
    1
    		+
    1
    		 =
    5
    2x2y2z2

    ⇒ 
    4
    		 +
    4
    		+
    4
    		 =
    4 × 5
    2x2y2z2

    ⇒ 
    2
    		 +
    2
    		+
    2
    		 = 10
    xyz

    Correct Option: C

    3 − 5x
    		 +
    3 − 5y
    		 +
    3 − 5z
    		 = 0
    2x2y2z

    ⇒ 
    3
    		−
    5x
    		 +
    3
    		−
    5y
    		 +
    3
    		 −
    5z
    		 = 0
    2x2x2y2y2z2z

    ⇒ 
    3
    		+
    3
    		+
    3
    		 −
    5
    		 −
    5
    		 −
    5
    		 = 0
    2x2y2z222

    ⇒ 
    3
    		+
    3
    		+
    3
    		 =
    3 × 5
    2x2y2z2

    ⇒ 
    1
    		 +
    1
    		+
    1
    		 =
    5
    2x2y2z2

    ⇒ 
    4
    		 +
    4
    		+
    4
    		 =
    4 × 5
    2x2y2z2

    ⇒ 
    2
    		 +
    2
    		+
    2
    		 = 10
    xyz