466. If	2x − y	=	1	, then value of	3x − y	is :
     	x + 2y		2		3x + y

1. 1. 1

      5

   
   
   

   2. 3

      5

   
   
   

   3. 4

      5

   
   
   

   4. 1

   
   
   

---

1. View Hint View Answer Discuss in Forum

   2x − y	=	1
   x + 2y		2

   
   ⇒  4x – 2y = x + 2y
   ⇒  3x = 4y
   

   ⇒	x	=	4
   	y		3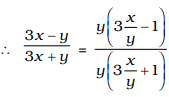

   
   
   

   =	3 ×	4	− 1
   		3
   	3 ×	4	+ 1
   		3

   
   

   =	4 − 1	=	3
   	4 + 1		5

   Correct Option: B

   2x − y	=	1
   x + 2y		2

   
   ⇒  4x – 2y = x + 2y
   ⇒  3x = 4y
   

   ⇒	x	=	4
   	y		3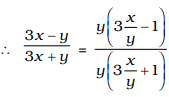

   
   
   

   =	3 ×	4	− 1
   		3
   	3 ×	4	+ 1
   		3

   
   

   =	4 − 1	=	3
   	4 + 1		5

---

467. If a and b be positive integers such that a² – b²= 19, then the value of a is

1. 1. 19

   
   
   

   2. 20

   
   
   

   3. 9

   
   
   

   4. 10

   
   
   

---

1. View Hint View Answer Discuss in Forum

   Tricky approach
   a² – b² = 19
   ⇒  10² – 9² = 19
   ⇒  a = 10

   Correct Option: D

   Tricky approach
   a² – b² = 19
   ⇒  10² – 9² = 19
   ⇒  a = 10

---

---

468. √3 + x + √3 − x	= 2 then x is equal to
     √3 + x − √3 − x

1. 1. 5

      12

   
   
   

   2. 12

      5

   
   
   

   3. 5

      7

   
   
   

   4. 7

      5

      
      

   
   
   

---

1. View Hint View Answer Discuss in Forum

   √3 + x + √3 − x	=	2
   √3 + x − √3 − x		1

   
   By componendo and dividendo,
   

   ⇒	2√3 + x	=	2 + 1	= 3
   	2√3 − x		2 − 1

   
   Squaring on both sides, we get
   

   3 + x	= 9
   3 − x

   
   ⇒  3 + x = 27 – 9x
   ⇒  9x + x = 27 – 3 = 24
   

   ⇒  x =	24	=	12
   	10		5

   Correct Option: B

   √3 + x + √3 − x	=	2
   √3 + x − √3 − x		1

   
   By componendo and dividendo,
   

   ⇒	2√3 + x	=	2 + 1	= 3
   	2√3 − x		2 − 1

   
   Squaring on both sides, we get
   

   3 + x	= 9
   3 − x

   
   ⇒  3 + x = 27 – 9x
   ⇒  9x + x = 27 – 3 = 24
   

   ⇒  x =	24	=	12
   	10		5

---

469. If   x +	1	= 5, then	2x	is equal to
     	x		3x2 − 5x + 3

1. 1. 5

   
   
   

   2. 1

      5

   
   
   

   3. 3

   
   
   

   4. 1

      3

   
   
   

---

1. View Hint View Answer Discuss in Forum

   x +	1	= 5
   	x

   
   ⇒  x² – 5x + 1 = 0
   ⇒  3x² – 15x + 3 = 0
   

   ∴	2x	=	2x
   	3x2 – 5x + 3		15x – 5x

   
   

   =	2x	=	1
   	10x		5

   Correct Option: B

   x +	1	= 5
   	x

   
   ⇒  x² – 5x + 1 = 0
   ⇒  3x² – 15x + 3 = 0
   

   ∴	2x	=	2x
   	3x2 – 5x + 3		15x – 5x

   
   

   =	2x	=	1
   	10x		5

---

---

470. If   x =	√3	, then the value of		√1 + x + √1 − x		is
     	2			√1 + x − √1 − x

1. 1. −√3

   
   
   

   2. –1

   
   
   

   3. 1

   
   
   

   4. √3

   
   
   

---

1. View Hint View Answer Discuss in Forum

   x =	√3	⇒	1	=	2
   	2		x		√3

   
   By componendo and dividendo,
   

   1 + x	=	2 + √3
   1 − x		2 − √3

   
   

   ⇒	1 + x	=	2 + √3	×	2 + √3
   	1 − x		2 − √3		2 + √3

   
   

   =	(2 + √3)2	=	(2 + √3)2
   	(2 − 3 √3)(2 + √3)		4 − 3

   
   

   ⇒	1 + x	= (2 + √3)2
   	1 − x

   
   

   ∴	√1 + x	=	2 + √3
   	√1 − x		1

   
   By componendo and dividendo
   

   √1 + x + √1 − x	=	2 + √3 + 1
   √1 + x − √1 − x		2 + √3 − 1

   
   

   =	3 + √3	=	√3( √3 + 1)	= √3
   	√3 + 1		√3 + 1

   Correct Option: D

   x =	√3	⇒	1	=	2
   	2		x		√3

   
   By componendo and dividendo,
   

   1 + x	=	2 + √3
   1 − x		2 − √3

   
   

   ⇒	1 + x	=	2 + √3	×	2 + √3
   	1 − x		2 − √3		2 + √3

   
   

   =	(2 + √3)2	=	(2 + √3)2
   	(2 − 3 √3)(2 + √3)		4 − 3

   
   

   ⇒	1 + x	= (2 + √3)2
   	1 − x

   
   

   ∴	√1 + x	=	2 + √3
   	√1 − x		1

   
   By componendo and dividendo
   

   √1 + x + √1 − x	=	2 + √3 + 1
   √1 + x − √1 − x		2 + √3 − 1

   
   

   =	3 + √3	=	√3( √3 + 1)	= √3
   	√3 + 1		√3 + 1

---