Height and Distance

Height and Distance

Easy Questions
Moderate Questions
Difficult Questions
Height and Distance Tutorial

Aptitude

Simplification
Number System
Fractions
Elementary Algebra
LCM and HCF
Approximation
Unitary Method
Linear Equation
Quadratic Equation
Discount
Average
Surds and Indices
Percentage
Square root and cube root
Order of Magnitude
Work and Wages
Odd Man Out and Series
Algebra
Profit and Loss
Stocks and Shares
True Discount
Ratio, Proportion
Partnership
Alligation or Mixture
Time and Work
Pipes and Cistern
Speed, Time and Distance
Problem on Trains
Height and Distance
Banker's Discount
Boats and Streams
Races and games
Problems on Ages
Clocks and Calendars
Simple interest
Compound Interest
Sets and Functions
Area and Perimeter
Volume and Surface Area of Solid Figures
Sequences and Series
Plane Geometry
Logarithm
Probability
Permutation and Combination
Statistics
Mensuration
Trigonometry
Aptitude miscellaneous
  1. The angle of elevation of the top of a hill from each of the vertices A, B, C of a horizontal triangle is α. The height of the hill is :








  1. View Hint View Answer Discuss in Forum

    As per the given details of above question , we have
    The distance of the foot from each vertex = h cotα.
    ∴ The foot is at the circumcentre of the triangle.
    ∴ R = h cotα

    Correct Option: B

    As per the given details of above question , we have
    The distance of the foot from each vertex = h cotα.
    ∴ The foot is at the circumcentre of the triangle.
    ∴ R = h cotα

    ∴ R = h tanα = atanα = a tanα . cosecα .
    2sinα2

  1. The angle of elevation of the top of an unfinished tower at a point distant 120 m from its base is 45°. If the elevation of the top at the same point is to be 60°, the tower must be raised to a height :








  1. View Hint View Answer Discuss in Forum

    Let us draw the figure from the given question.
    Given :- OB = h + x

    h + x = tan 60° = √3
    120

    h + x= √3 (120) .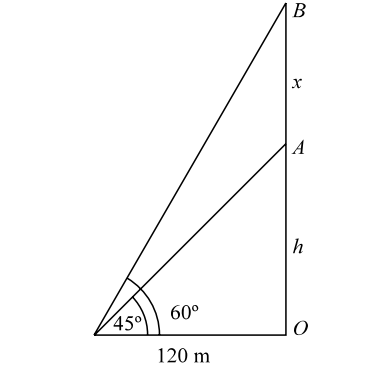


    Correct Option: B

    Let us draw the figure from the given question.
    Given :- OB = h + x
    In figure , we have

    h + x = tan 60° = √3
    120

    h + x= √3 (120) ...........( 1 )

    Also, h = tan 45° = 1 .
    120

    ∴ h = 120 m

    From eq. ( 1 ) ,
    ∴ h + x = 120 + x = 120 √3
    ∴ x = 120 ( √3 - 1 ) m .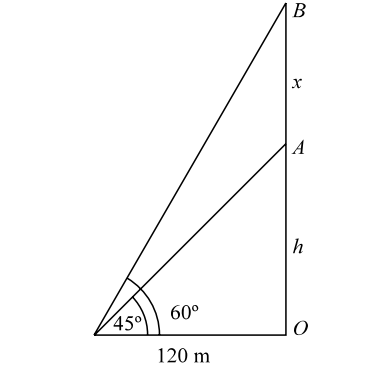


  1. A person standing on the bank of a river observes that the angle subtended by a tree on the opposite bank is 60°. When he retires 40 m from the bank, he finds the angle to be 30°. The breadth of the river is :








  1. View Hint View Answer Discuss in Forum

    Let us draw the figure from the given question.
    Let, OA denote the breadth of the river.
    Given :- AB = 40 m


    Correct Option: C

    Let us draw the figure from the given question.
    Let, OA denote the breadth of the river.
    Given :- AB = 40 m
    In triangle OAP , we have

    OP= tan60° = √3
    OA

    ∴OP = √3 OA.

    Also, In triangle OBP , we have OP = tan 30° = 1
    OA + 40√3

    ∴ OA + 40 = √3 OP = √3 ( √3 OA ) = 3 OA.

    ⇒ 2OA = 40 ⇒ OA = 20 m.

    Hence , the breadth of the river = 20 m .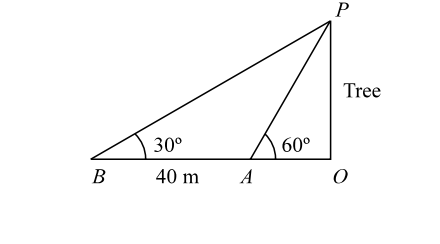


  1. The angle of elevation of the top of a tower at a point G on the ground is 30°. On walking 20 m towards the tower, the angle of elevation becomes 60°. The height of the tower is equal to :








  1. View Hint View Answer Discuss in Forum

    Let us draw the figure from the given question.
    Let, AB = h be the height of the tower. Let, GA = x.


    Correct Option: D

    Let us draw the figure from the given question.
    Let, AB = h be the height of the tower. Let, GA = x and GH = 20 m.
    In triangle BGA ,

    Then, h = tan30° = 1 .
    x√3

    ∴ h = x ......... ( 1 )
    √3

    In triangle BHA , Also,
    h
    		 = tan60° = √3 .
    x - 20

    ∴ h = √3 ( x - 20 )

    ∴ x = √3 ( x - 20 ) [ using ( 1 ) ]
    √3

    ⇒ x = 3 ( x - 20 ) = 3x - 60

    ⇒ 2x = 60 ⇒ x = 30 .
    Putting the value of x in eq. ( 1 ) , we get
    ∴ h = 30 = 10 √3 m .
    √3


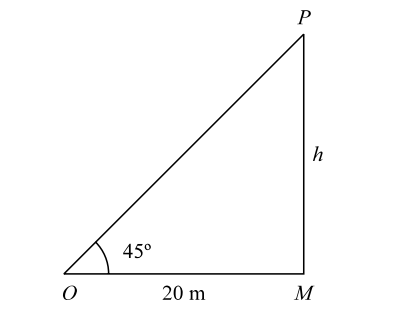
  1. The angle of elevation of the top of a tower from a point 20 m away from its base is 45°. The height of the tower is :








  1. View Hint View Answer Discuss in Forum

    Let us draw the figure from the given question.
    Let us assume the angle of elevation ∠MŌP = 45°.
    Given :- MO = 20 m
    In triangle MOP ,

    tan45° = PM
    MO

    Clearly,h = tan45 = 1
    20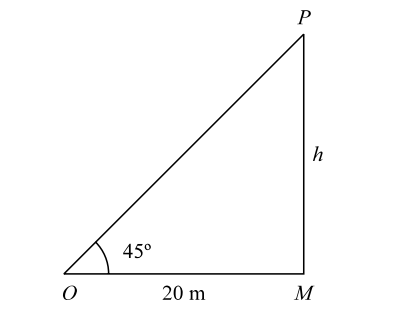


    Correct Option: B

    From the given figure , we can see that
    Let us assume the angle of elevation ∠MŌP = 45°. and The height of the tower = h
    Given :- MO = 20 m
    In triangle MOP ,

    tan45° = PM
    MO

    ⇒ h = tan45° = 1
    20

    ∴ h = 20 m