Mensuration
- The base of a right prism, whose height is 2 cm, is a square. If the total surface area of the prism is 10 cm², then its volume is :
-
View Hint View Answer Discuss in Forum
Total surface area of prism = Perimeter of base × height + 2 × area of base
10 = 4x × 2 + 2x²
where x = side of square
⇒ x² + 4x – 5 = 0
⇒ x² + 5x – x – 5 = 0
⇒ x (x + 5) –1 (x + 5) = 0
⇒ (x – 1) (x + 5) = 0
⇒ x = 1 because x ≠ –5
∴ Volume of prism = Area of base × height = 1 × 1 × 2 = 2 cu.cm.Correct Option: C
Total surface area of prism = Perimeter of base × height + 2 × area of base
10 = 4x × 2 + 2x²
where x = side of square
⇒ x² + 4x – 5 = 0
⇒ x² + 5x – x – 5 = 0
⇒ x (x + 5) –1 (x + 5) = 0
⇒ (x – 1) (x + 5) = 0
⇒ x = 1 because x ≠ –5
∴ Volume of prism = Area of base × height = 1 × 1 × 2 = 2 cu.cm.
- The total surface area of a right pyramid on a square base of side 10 cm with height 12 cm is :
-
View Hint View Answer Discuss in Forum
Slant height of pyramid = √5² + 12² = √25 + 144
= √169 = 13 cm.Lateral surface area of pyramid = 1 × perimeter of base × slant height 2 = 1 × 4 × 10 × 13 = 260 sq. cm. 2
Area of base = 10 × 10 = 100 sq.cm.
∴ Total surface area = 260 + 100 = 360 sq.cm.Correct Option: B
Slant height of pyramid = √5² + 12² = √25 + 144
= √169 = 13 cm.Lateral surface area of pyramid = 1 × perimeter of base × slant height 2 = 1 × 4 × 10 × 13 = 260 sq. cm. 2
Area of base = 10 × 10 = 100 sq.cm.
∴ Total surface area = 260 + 100 = 360 sq.cm.
- The area of the largest sphere (in cm²) that can be drawn inside a square of side 18 cm is
-
View Hint View Answer Discuss in Forum
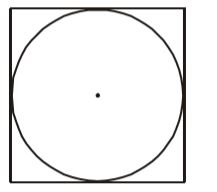
Radius of the largest sphere = 18 = 9 cm. 2
∴ Area of sphere = 4πr² = 4π × 9 × 9 = 972p sq. cm.Correct Option: A

Radius of the largest sphere = 18 = 9 cm. 2
∴ Area of sphere = 4πr² = 4π × 9 × 9 = 972p sq. cm.
- The diameter of a sphere is twice the diameter of another sphere. The surface area of the first sphere is equal to the volume of the second sphere. The magnitude of the radius of the first sphere is
-
View Hint View Answer Discuss in Forum
Radius of first sphere = 2r cm.
Radius of second sphere = r cm.
According to the question,4π(2r)² = 4 πr³ 3 ⇒ 4πr² = 4 πr³ 3
⇒ 12 = r
∴ Radius of first sphere = 24 cm.Correct Option: B
Radius of first sphere = 2r cm.
Radius of second sphere = r cm.
According to the question,4π(2r)² = 4 πr³ 3 ⇒ 4πr² = 4 πr³ 3
⇒ 12 = r
∴ Radius of first sphere = 24 cm.
- The lateral surface area of frustum of a right circular cone, if the area of its base is 16π cm² and the diameter of circular upper surface is 4 cm and slant height is 6 cm, will be
-
View Hint View Answer Discuss in Forum
According to the question,
πR² = 16π
⇒ R² = 16
⇒ R = 16 = 4 cm.
∴ Required area = π(R + r)l
= π(4 + 2) × 6 = 36π sq. cm.Correct Option: C
According to the question,
πR² = 16π
⇒ R² = 16
⇒ R = 16 = 4 cm.
∴ Required area = π(R + r)l
= π(4 + 2) × 6 = 36π sq. cm.

