171. The radius of base and slant height of a cone are in the ratio 4 : 7. If its curved surface area is 792 cm², then the radius (in cm) of its base is [Use π = 22/7]
     

1. 1. 8
      

   
   
   

   2. 12
      

   
   
   

   3. 14
      

   
   
   

   4. 16

   
   
   

---

1. View Hint View Answer Discuss in Forum

   Let radius = 4x cm and slant height (l) = 7x cm
   

   ∴ πrl =	22	× 4x × 7x = 792
   	12

   
   

   ⇒ x² =	792 × 7	= 9
   	22 × 4 × 7

   
   ∴ x = 3
   ∴ Radius = 4 × 3 = 12 cm

   Correct Option: B

   Let radius = 4x cm and slant height (l) = 7x cm
   

   ∴ πrl =	22	× 4x × 7x = 792
   	12

   
   

   ⇒ x² =	792 × 7	= 9
   	22 × 4 × 7

   
   ∴ x = 3
   ∴ Radius = 4 × 3 = 12 cm

---

172. A semi-circular sheet of metal of diameter 28 cm is bent into an open conical cup. The depth of the cup is approximately
     

1. 1. 11 cm
      

   
   
   

   2. 12 cm
      

   
   
   

   3. 13 cm
      

   
   
   

   4. 14 cm

   
   
   

---

1. View Hint View Answer Discuss in Forum

   
   
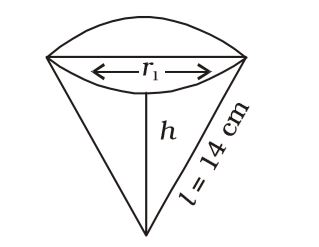
   Length (ACB) of semi-circular sheet = πr
   

   =	22	× 14 = 44 cm.
   	7

   
   Slant height of the cone = 14 cm.
   

   Circumference of the base of the cone = 2πr1 =	44	r1
   	7

   
   

   ⇒ 44 =	44	r1 ⇒ r1 = 7 cm.
   	7

   
   ∴ h = √l² - r₁² = √14² - 7²
   = 7√3 cm. = 7 × 1.732 ≈ 12cm.

   Correct Option: B

   
   
   Length (ACB) of semi-circular sheet = πr
   

   =	22	× 14 = 44 cm.
   	7

   
   Slant height of the cone = 14 cm.
   

   Circumference of the base of the cone = 2πr1 =	44	r1
   	7

   
   

   ⇒ 44 =	44	r1 ⇒ r1 = 7 cm.
   	7

   
   ∴ h = √l² - r₁² = √14² - 7²
   = 7√3 cm. = 7 × 1.732 ≈ 12cm.

---

---

173. The area of the four walls of a room is 660 m² and its length is twice its breadth. If the height of the room is 11 m, then area of its floor (in m²) is
     

1. 1. 120
      

   
   
   

   2. 150
      

   
   
   

   3. 200
      

   
   
   

   4. 330

   
   
   

---

1. View Hint View Answer Discuss in Forum

   Let Breadth of room = x metre Length = 2x metre
   ∴ Area of four walls = 2 × h (l + b)
   ⇒ 660 = 2 × 11 (2x + x) = 22 × 3x = 66 x
   

   ⇒ x =	660	= 10
   	66

   
   ∴ Area of floor = 2x² = 2 × 10² = 200 sq.metre

   Correct Option: C

   Let Breadth of room = x metre Length = 2x metre
   ∴ Area of four walls = 2 × h (l + b)
   ⇒ 660 = 2 × 11 (2x + x) = 22 × 3x = 66 x
   

   ⇒ x =	660	= 10
   	66

   
   ∴ Area of floor = 2x² = 2 × 10² = 200 sq.metre

---

174. A right angled sector of radius r cm is rolled up into a cone in such a way that the two binding radii are joined together. Then the curved surface area of the cone is
     

1. 1. πr² cm²
      

   
   
   

   2. 4πr² cm²
      

   
   
   

   3. 	πr²	cm²
      	4

   
   
   

   4. 2πr² cm²

   
   
   

---

1. View Hint View Answer Discuss in Forum

   Radius of the base (r1) =	r
   	4

   
   Slant height = r
   

   ∴ Curved surface area = πr1l =	πr²	cm²
   	4

   Correct Option: C

   Radius of the base (r1) =	r
   	4

   
   Slant height = r
   

   ∴ Curved surface area = πr1l =	πr²	cm²
   	4

---

---

175. The radius of the base of a conical tent is 16 metre. If 427(3/7)sq. metre canvas is required to construct the tent, then the slant height of the tent is : (Take π = 22/7)
     

1. 1. 17 metre
      

   
   
   

   2. 15 metre
      

   
   
   

   3. 19 metre
      

   
   
   

   4. 8.5 metre

   
   
   

---

1. View Hint View Answer Discuss in Forum

   Curved surface area of cone = πrl
   

   ∴	22	× 16 × l =	2992
   	7		7

   
   ⇒ 22 × 16 × l = 2992
   

   ⇒ l =	2992	= 8.5 metre
   	22 × 16

   Correct Option: D

   Curved surface area of cone = πrl
   

   ∴	22	× 16 × l =	2992
   	7		7

   
   ⇒ 22 × 16 × l = 2992
   

   ⇒ l =	2992	= 8.5 metre
   	22 × 16

---