Mensuration
- If 64 buckets of water are removed from a cubical shaped water tank completely filled with water, 1/3 of the tank remains filled with water. The length of each side of the tank is 1.2 m. Assuming that all buckets are of the same measure, then the volume (in litres) of water contained by each bucket is
-
View Hint View Answer Discuss in Forum
Volume of tank = (1.2)³ cubic metre = 1.728 cubic metre
∴ 64 × Volume of 1 bucket= 2 × 1.728 cubic metre 3
∴ Volume of 1 bucket= 
1.728 × 2 
cubic metre 3 × 64
= 0.018 cubic metre = (0.018 × 1000) litres = 18 litresCorrect Option: D
Volume of tank = (1.2)³ cubic metre = 1.728 cubic metre
∴ 64 × Volume of 1 bucket= 2 × 1.728 cubic metre 3
∴ Volume of 1 bucket= 
1.728 × 2 
cubic metre 3 × 64
= 0.018 cubic metre = (0.018 × 1000) litres = 18 litres
- A conical cup is filled with icecream. The ice-cream forms a hemispherical shape on its open top. The height of the hemispherical part is 7 cm. The radius of the hemispherical part equals the height of the cone. Then the volume of the ice-cream is (π = 22/7)
-
View Hint View Answer Discuss in Forum
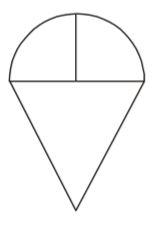
Volume of hemisphere = 2 πr³ 3
Where r = radius = 7 cm.= 
2 × 22 × 7 × 7 × 7 
cu.cm. 3 7 Volume of conical part = 1 πr²h 3
[∵ r = h]= 
1 × 22 × 7 × 7 × 7 
cu.cm. 3 7 ∴ Volume of ice-cream = 2 × 22 × 7³ + 1 × 22 × 7³ = 22 × 7³ = 22 × 7² = 1078 cu.cm. 3 7 3 7 7 Correct Option: A

Volume of hemisphere = 2 πr³ 3
Where r = radius = 7 cm.= 
2 × 22 × 7 × 7 × 7 
cu.cm. 3 7 Volume of conical part = 1 πr²h 3
[∵ r = h]= 
1 × 22 × 7 × 7 × 7 
cu.cm. 3 7 ∴ Volume of ice-cream = 2 × 22 × 7³ + 1 × 22 × 7³ = 22 × 7³ = 22 × 7² = 1078 cu.cm. 3 7 3 7 7
- A hollow sphere of internal and external diameters 6 cm and 10 cm respectively is melted into a right circular cone of diameter 8 cm. The height of the cone is
-
View Hint View Answer Discuss in Forum
Volume of material of hollow sphere = 4 π (r1³ - r2³) 3 = 4 π (5³ - 3³) 3 = 4 π (125 - 27) 3 = 4 π × 98 cu.cm. 3 Volume of cone = 1 πr²h 3 = 1 π × 4² × h 3 ∴ 1 π × 16 × h = 4 × π × 98 3 3
⇒4h = 98⇒ h = 98 = 24.5 cm. 4 Correct Option: C
Volume of material of hollow sphere = 4 π (r1³ - r2³) 3 = 4 π (5³ - 3³) 3 = 4 π (125 - 27) 3 = 4 π × 98 cu.cm. 3 Volume of cone = 1 πr²h 3 = 1 π × 4² × h 3 ∴ 1 π × 16 × h = 4 × π × 98 3 3
⇒4h = 98⇒ h = 98 = 24.5 cm. 4
- Each edge of a regular tetrahedron is 4 cm. Its volume (in cubic cm) is
-
View Hint View Answer Discuss in Forum
Volume of the tetrahedron = a³ where; a = edge = 4 cm 6√2 = 4 × 4 × 4 = 16√2 cu.cm. 6√2 3 Correct Option: C
Volume of the tetrahedron = a³ where; a = edge = 4 cm 6√2 = 4 × 4 × 4 = 16√2 cu.cm. 6√2 3
- A flask in the shape of a right circular cone of height 24 cm is filled with water. The water is poured in right circular cylindrical flask whose radius is 1/3 rd of radius of the base of the circular cone. Then the height of the water in the cylindrical flask is
-
View Hint View Answer Discuss in Forum
Radius of the base of conical shape = r cm (let)
Radius of base of cylinder = r cm. 3 Volume of water = Volume of cone = 1 πr²h = 1 πr² × 24 3 3
= 8πr² cu. cm.
∴ Volume of cylinder = πR²H= π × 
r 
² H = πr²H cu.cm. 3 9 ∴ πr²H = 8πr² 9
⇒ H = 9 × 8 = 72 cmCorrect Option: D
Radius of the base of conical shape = r cm (let)
Radius of base of cylinder = r cm. 3 Volume of water = Volume of cone = 1 πr²h = 1 πr² × 24 3 3
= 8πr² cu. cm.
∴ Volume of cylinder = πR²H= π × 
r 
² H = πr²H cu.cm. 3 9 ∴ πr²H = 8πr² 9
⇒ H = 9 × 8 = 72 cm

