Mensuration
- The base area of a right pyramid is 57 sq. units and height is 10 units. Then the volume of the pyramid is
-
View Hint View Answer Discuss in Forum
Area of the base of pyramid = 57 sq. units
Height = 10 units∴ Volume of pyramid = 1 × Area of base × height 3 = 
1 × 57 × 10 
cu.cm. 3
= 190 cu. unitsCorrect Option: A
Area of the base of pyramid = 57 sq. units
Height = 10 units∴ Volume of pyramid = 1 × Area of base × height 3 = 
1 × 57 × 10 
cu.cm. 3
= 190 cu. units
- If a cone is divided into two parts by drawing a plane through the midpoints of its axis, then the ratio of the volume of the two parts of the cone is
-
View Hint View Answer Discuss in Forum
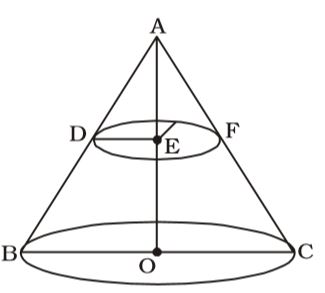
Volume of larger cone = 1 πr²h 3
∆ADE ~ ∆ABO∴ DE = AE BO AO ⇒ = h ⇒ DE = DE 2 r r h 2 Volume of cone ADF = 1 π 
r 
² × h 3 2 2 = 1 πr²h cu. units 24 Volume of remaining part = 1 πr²h - 1 πr²h 3 24 = πr²h 
1 - 3 
3 24 = πr²h 
8 - 1 
24 = 7 πr²h cu.units 24 ∴ Required ratio = 1 πr²h : 7 πr²h 24 24
= 1 : 7Correct Option: C

Volume of larger cone = 1 πr²h 3
∆ADE ~ ∆ABO∴ DE = AE BO AO ⇒ = h ⇒ DE = DE 2 r r h 2 Volume of cone ADF = 1 π 
r 
² × h 3 2 2 = 1 πr²h cu. units 24 Volume of remaining part = 1 πr²h - 1 πr²h 3 24 = πr²h 
1 - 3 
3 24 = πr²h 
8 - 1 
24 = 7 πr²h cu.units 24 ∴ Required ratio = 1 πr²h : 7 πr²h 24 24
= 1 : 7
- A right circular cylinder is partially filled with water. Two iron spherical balls are completely immersed in the water so that the height of the water in the cylinder rises by 4 cm. If the radius of one ball is half of the other and the diameter of the cylinder is 18 cm., then the radii of the spherical balls are
-
View Hint View Answer Discuss in Forum
Volume of both spheres = Volume of water raised in the cylinder
= π × 9² × 4 = 324π cu. cm.
Radius of first sphere = r cm.Radius of second sphere = r cm 2 ∴ 4 π 
r³ + r³ 
= 324π 3 8 ⇒ 
8r³ + r³ 
= 324 × 3 8 4 ⇒ 9r³ = 243 8 ⇒ r³ = 243 × 8 = 216 9 ∴ r = 216 = 6 cm. 3
∴ Radius of second sphere = 3 cm.Correct Option: C
Volume of both spheres = Volume of water raised in the cylinder
= π × 9² × 4 = 324π cu. cm.
Radius of first sphere = r cm.Radius of second sphere = r cm 2 ∴ 4 π 
r³ + r³ 
= 324π 3 8 ⇒ 
8r³ + r³ 
= 324 × 3 8 4 ⇒ 9r³ = 243 8 ⇒ r³ = 243 × 8 = 216 9 ∴ r = 216 = 6 cm. 3
∴ Radius of second sphere = 3 cm.
- The radii of two cylinders are in the ratio of 3 : 2 and their heights are in the ratio 3 : 7. The ratio of their volumes is :
-
View Hint View Answer Discuss in Forum
r1 = 3 : h1 = 3 r2 2 h2 7 ∴ V1 = πr1²h1 = 
r1 
² × h1 V2 πr2²h2 r2 h2 = 
3 
² × 3 2 7 = 9 × 3 = 27 = 27 : 28 4 7 28 Correct Option: D
r1 = 3 : h1 = 3 r2 2 h2 7 ∴ V1 = πr1²h1 = 
r1 
² × h1 V2 πr2²h2 r2 h2 = 
3 
² × 3 2 7 = 9 × 3 = 27 = 27 : 28 4 7 28
- If the volumes of two right circular cones are in the ratio 1 : 4 and their diameters of bases are in the ratio 4 : 5, then their heights will be in the ratio :
-
View Hint View Answer Discuss in Forum
d1 = r1 = 4 d2 r2 5 ∴ = 1 πr1²h1 V1 3 V2 1 πr2²h2 3 ⇒ V1 = 
r1 
² × h1 V2 r2 h2 ⇒ V1 = 
4 
² × h1 V2 5 h2 ⇒ h1 = 1 × 5 × 5 = 25 = 25 : 64 h2 4 4 × 4 64 Correct Option: D
d1 = r1 = 4 d2 r2 5 ∴ = 1 πr1²h1 V1 3 V2 1 πr2²h2 3 ⇒ V1 = 
r1 
² × h1 V2 r2 h2 ⇒ V1 = 
4 
² × h1 V2 5 h2 ⇒ h1 = 1 × 5 × 5 = 25 = 25 : 64 h2 4 4 × 4 64

