Mensuration
- In an isosceles triangle, the length of each equal side is twice the length of the third side. The ratio of areas of the isosceles triangle and an equilateral triangle with same perimeter is
-
View Hint View Answer Discuss in Forum
Let the third side of isosceles triangle be x units and side of equilateral triangle be y units. According to the question, 2x + 2x + x = 3y
⇒ 5x = 3y ..... (i)Area of equilateral triangle = √3 y² 4 AB = 2x ; BD = x units 2
∴ AD = √AB² - BD²= √4x² - x² 4 = √ 16x² - x² = √15 x 4 2 Area of isosceles triangle ABC = 1 × x × √15 x = √15 x² 2 2 4 = √15 × 
3 y 
² 4 5 = 9√15 y² 100 ∴ Required ratio = 9√15 y² : √3 y² = 36√5 : 100 100 4 Correct Option: C
Let the third side of isosceles triangle be x units and side of equilateral triangle be y units. According to the question, 2x + 2x + x = 3y
⇒ 5x = 3y ..... (i)Area of equilateral triangle = √3 y² 4 AB = 2x ; BD = x units 2
∴ AD = √AB² - BD²= √4x² - x² 4 = √ 16x² - x² = √15 x 4 2 Area of isosceles triangle ABC = 1 × x × √15 x = √15 x² 2 2 4 = √15 × 
3 y 
² 4 5 = 9√15 y² 100 ∴ Required ratio = 9√15 y² : √3 y² = 36√5 : 100 100 4
- The radius of the incircle of an equilateral DABC of side 23 units is x cm. The value of x is :
-
View Hint View Answer Discuss in Forum
In-radius (x) = Side = 2√3 = 1 cm 2√3 2√3 Correct Option: C
In-radius (x) = Side = 2√3 = 1 cm 2√3 2√3
- The four sides of a quadrilateral are in the ratio of 2 : 3 : 4 : 5 and its perimeter is 280 metre. The length of the longest side is :
-
View Hint View Answer Discuss in Forum
Sides of quadrilateral
= 2x, 3x, 4x and 5x metre According to the question, 2x + 3x + 4x + 5x = 280⇒ 14x = 280 ⇒ x = 280 = 20 14
∴ Largest side = 5x = 5 × 20 = 100 cm.
OR
a : b : c : d = 2 : 3 : 4 : 5
Sum of the terms of ratio = 2 + 3 + 4 + 5 = 14 a + b + c + d = 280 metre∴ Largest side = 5 × 280 = 100 metre 14 Correct Option: A
Sides of quadrilateral
= 2x, 3x, 4x and 5x metre According to the question, 2x + 3x + 4x + 5x = 280⇒ 14x = 280 ⇒ x = 280 = 20 14
∴ Largest side = 5x = 5 × 20 = 100 cm.
OR
a : b : c : d = 2 : 3 : 4 : 5
Sum of the terms of ratio = 2 + 3 + 4 + 5 = 14 a + b + c + d = 280 metre∴ Largest side = 5 × 280 = 100 metre 14
- If x is the area, y is the circumference and z is the diameter of
circle then the value of x/yz is
-
View Hint View Answer Discuss in Forum
Diameter of circle = z = 2r
⇒ r = z units 2 Circumference = 2πr = 2π × z units 2
⇒ y = πz units
Area = πr²⇒ x = π 
z 
² 2 ⇒ x = πz² sq.cm. 4
∴ = πz² = = 1 : 4 x 4 1 yz πz × z 4 Correct Option: B
Diameter of circle = z = 2r
⇒ r = z units 2 Circumference = 2πr = 2π × z units 2
⇒ y = πz units
Area = πr²⇒ x = π 
z 
² 2 ⇒ x = πz² sq.cm. 4
∴ = πz² = = 1 : 4 x 4 1 yz πz × z 4
- The lengths of diagonals of a rhombus are 24 cm and 10 cm the perimeter of the rhombus (in cm.) is :
-
View Hint View Answer Discuss in Forum
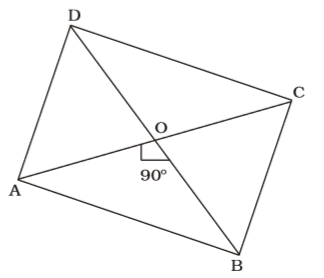
The diagonals of a rhombus bisect each other at right angles.
Let AC = 24 cm.
∴ AO = 12 cm.
BD = 10 cm.
∴ BO = 5 cm.
In ∆AOB,
AB = √OA² + OB²
= √12² + 5²+
= √144 + 25 = √169
= 13 cm.
∴ Perimeter of rhombus = 4 × 13 = 52 cm.Correct Option: A

The diagonals of a rhombus bisect each other at right angles.
Let AC = 24 cm.
∴ AO = 12 cm.
BD = 10 cm.
∴ BO = 5 cm.
In ∆AOB,
AB = √OA² + OB²
= √12² + 5²+
= √144 + 25 = √169
= 13 cm.
∴ Perimeter of rhombus = 4 × 13 = 52 cm.
-
-