Mensuration
- The base of a right prism is a quadrilateral ABCD. Given that AB = 9 cm, BC = 14 cm, CD = 13 cm, DA = 12 cm and ∠DAB = 90°. If the volume of the prism be 2070 cm³, then the area of the lateral surface is
-
View Hint View Answer Discuss in Forum
Area of base = Area of ∆ABD + Area of ∆BCD
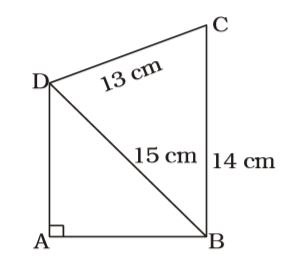
In, ∆ABD
BD = √AB² + AD² = √9² + 12²
= √81 + 144 = √225 = 15 cm
Area of ∆ABDSemi-perimeter (s) = 13 + 14 + 15 = 42 = 21 2 2
∴ Area of ∆BCD
= √s(s - a)(s - b)(s - c)
= √21(21 - 13)(21 - 14)(21 - 15)
= √21 × 8 × 7 × 6
= 21 × 4 = 84 sq. cm
Area of quadrilateral ABCD = 54 + 84 = 138 sq, cm∴ Height of prism = Volume = 2070 = 15 cm. Area of base 138
Perimeter of base = (9 + 14 + 13 +12) cm = 48 cm
∴ Area of lateral surfaces = perimeter × height
= 48 × 15 = 720 sq. cm.Correct Option: A
Area of base = Area of ∆ABD + Area of ∆BCD

In, ∆ABD
BD = √AB² + AD² = √9² + 12²
= √81 + 144 = √225 = 15 cm
Area of ∆ABDSemi-perimeter (s) = 13 + 14 + 15 = 42 = 21 2 2
∴ Area of ∆BCD
= √s(s - a)(s - b)(s - c)
= √21(21 - 13)(21 - 14)(21 - 15)
= √21 × 8 × 7 × 6
= 21 × 4 = 84 sq. cm
Area of quadrilateral ABCD = 54 + 84 = 138 sq, cm∴ Height of prism = Volume = 2070 = 15 cm. Area of base 138
Perimeter of base = (9 + 14 + 13 +12) cm = 48 cm
∴ Area of lateral surfaces = perimeter × height
= 48 × 15 = 720 sq. cm.
- An elephant of length 4 m is at one corner of a rectangular cage of size (16 m × 30 m) and faces towards the diagonally opposite corner. If the elephant starts moving towards the diagonally opposite corner it takes 15 seconds to reach this corner. Find the speed of the elephant.
-
View Hint View Answer Discuss in Forum
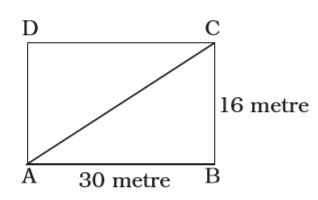
AC = √AB² + BC²
= √30² + 16²
= √900 + 256 = √1156 = 34 metre
Distance travelled by elephant = 34 – 4 = 30 metre∴ Speed of elephant = 30 = 2 m/sec. 15 Correct Option: B

AC = √AB² + BC²
= √30² + 16²
= √900 + 256 = √1156 = 34 metre
Distance travelled by elephant = 34 – 4 = 30 metre∴ Speed of elephant = 30 = 2 m/sec. 15
- A horse takes 2 (1/2) seconds to complete a round around a circular field. If the speed of the horse was 66 m/sec, then the radius of the field is, [Given π = 22/7]
-
View Hint View Answer Discuss in Forum
Distance covered in one revolution = Circumference of circular field = 2πr
Again,
speed of horse = 66 metre/secondTime = 5 Second 2 ∴ Distance covered = 66 × 5 = 165 metre 2
∴ 2πr = 165⇒ 2 × 22 × r = 165 7 ⇒ r = 165 × 7 = 26.25 metre 2 × 22 Correct Option: D
Distance covered in one revolution = Circumference of circular field = 2πr
Again,
speed of horse = 66 metre/secondTime = 5 Second 2 ∴ Distance covered = 66 × 5 = 165 metre 2
∴ 2πr = 165⇒ 2 × 22 × r = 165 7 ⇒ r = 165 × 7 = 26.25 metre 2 × 22
- Water is flowing at the rate of 5 km/h through a pipe of diameter 14 cm into a rectangular tank which is 50 m long, 44m wide. The time taken (in hours) for the rise in the level of water in the tank to be 7 cm is
-
View Hint View Answer Discuss in Forum
Water flowed by the pipe in 1 hour = πr²h
= 22 × 7 × 7 × 5000 = 77 m 7 100 × 100 Volume of expected water in the tank = 50 × 44 × 7 = 154 m³ 100 ∴ Required time = 154 = 2 hours 77 Correct Option: A
Water flowed by the pipe in 1 hour = πr²h
= 22 × 7 × 7 × 5000 = 77 m 7 100 × 100 Volume of expected water in the tank = 50 × 44 × 7 = 154 m³ 100 ∴ Required time = 154 = 2 hours 77
- The volume of a right circular cylinder is equal to the volume of that right circular cone whose height is 108 cm and diameter of base is 30 cm. If the height of the cylinder is 9 cm, the diameter of its base is
-
View Hint View Answer Discuss in Forum
Volume of the cone = 1 π × 15 × 15 × 108 3
Volume of cylinder = π × r² × 9 cm³
According to the question, π × r² × 9= 1 π × 15 × 15 × 108 3 ⇒ r² = 5 × 15 × 108 9
⇒ r² = 900 ⇒ r = 30
Diameter of base = 2r = 2 × 30 = 60 cm.Correct Option: B
Volume of the cone = 1 π × 15 × 15 × 108 3
Volume of cylinder = π × r² × 9 cm³
According to the question, π × r² × 9= 1 π × 15 × 15 × 108 3 ⇒ r² = 5 × 15 × 108 9
⇒ r² = 900 ⇒ r = 30
Diameter of base = 2r = 2 × 30 = 60 cm.

