-
If a cone is divided into two parts by drawing a plane through the midpoints of its axis, then the ratio of the volume of the two parts of the cone is
-
- 1 : 2
- 1 : 4
- 1 : 7
- 1 : 8
- 1 : 2
Correct Option: C
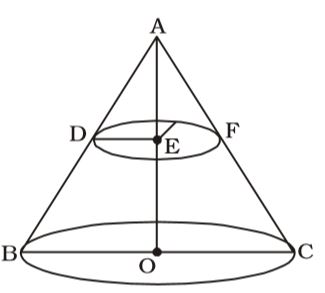
| Volume of larger cone = | πr²h | |
| 3 |
∆ADE ~ ∆ABO
| ∴ | = | ||
| BO | AO |
| ⇒ | = | ⇒ DE = | |||
| DE | 2 | r | |||
| r | h | 2 | |||
| Volume of cone ADF = | π |  |  | ² | × | |||
| 3 | 2 | 2 |
| = | πr²h cu. units | |
| 24 |
| Volume of remaining part = | πr²h - | πr²h | ||
| 3 | 24 |
| = πr²h |  | - |  | ||
| 3 | 24 |
| = πr²h |  |  | |
| 24 |
| = | πr²h cu.units | |
| 24 |
| ∴ Required ratio = | πr²h : | πr²h | ||
| 24 | 24 |
= 1 : 7

