Plane Geometry
- For a triangle ABC, D and E are two points on AB and AC such that AD = ( 1 / 4 )AB, AE = ( 1 / 4 )AC. If BC = 12 cm, then DE is
-
View Hint View Answer Discuss in Forum
As per the given in question , we draw a figure triangle ABC in which D and E are two points on AB and AC ,
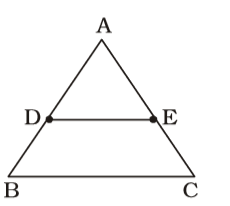
Given that , AD = 1 AB 4 AE = 1 AC and BC = 12 cm 4 AD = AB AE AC
∆ ADE ~ ∆ ABC∴ DE = 1 BC 4
Correct Option: C
As per the given in question , we draw a figure triangle ABC in which D and E are two points on AB and AC ,

Given that , AD = 1 AB 4 AE = 1 AC and BC = 12 cm 4 AD = AB AE AC
∆ ADE ~ ∆ ABC∴ DE = 1 BC 4 DE = 1 × 12 = 3 cm. 4
- In triangle ABC a straight line parallel to BC intersects AB and AC at D and E respectively. If AB = 2AD then DE : BC is
-
View Hint View Answer Discuss in Forum
According to question , we draw a figure of triangle ABC

From question , AB = 2 AD 1
∆ ADE ~ ∆ ABCAB = BC = 2 AD DE 1
Correct Option: C
According to question , we draw a figure of triangle ABC

From question , AB = 2 AD 1
∆ ADE ~ ∆ ABCAB = BC = 2 AD DE 1 ∴ DE = 1 = 1 : 2 BC 2
- In a ∆ ABC, D and E are two points on AB and AC respectively such that DE || BC, DE bisects the ∆ ABC in two equal areas. Then the ratio DB : AB is
-
View Hint View Answer Discuss in Forum
According to question , we draw a figure of triangle ABC in which D and E are two points on AB and AC respectively such that DE || BC ,

Given , ∆ ADE = ☐ BDEC
⇒ ∆ 2ADE = ∆ ABC
DE || BC
∴ ∆ ADE ~ ∆ ABC∴ ∆ ADE = 1 = AD² ∆ ABC 2 AB² ⇒ AB = √2 AD ⇒ AB - 1 = √2 - 1 AD ⇒ AB - AD = √2 - 1 AD ⇒ BD = √2 - 1 AD
Correct Option: C
According to question , we draw a figure of triangle ABC in which D and E are two points on AB and AC respectively such that DE || BC ,

Given , ∆ ADE = ☐ BDEC
⇒ ∆ 2ADE = ∆ ABC
DE || BC
∴ ∆ ADE ~ ∆ ABC∴ ∆ ADE = 1 = AD² ∆ ABC 2 AB² ⇒ AB = √2 AD ⇒ AB - 1 = √2 - 1 AD ⇒ AB - AD = √2 - 1 AD ⇒ BD = √2 - 1 AD ∴ BD = BD × AD = √2 - 1 AB AD AB √2
- In ∆ ABC, E and D are points on sides AB and AC respectively such that ∠ABC = ∠ADE. If AE = 3 cm, AD = 2 cm and EB = 2 cm, then length of DC is
-
View Hint View Answer Discuss in Forum
As per the given in question , we draw a figure triangle ABC
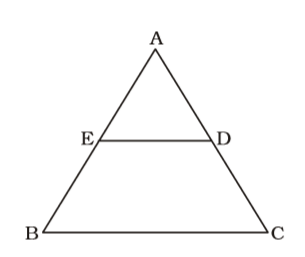
Here , AE = 3 cm, AD = 2 cm and EB = 2 cm,
In ∆ ADE and ∆ ABC,
∠ABC = ∠ADE
∠A = ∠A
∴ ∆ ABC ~ ∆ ADE∴ AB = BC = AC AD DE AE ∴ AB + EB = AC AD AE ⇒ 3 + 2 = AC 2 3 ⇒ 5 = AC 2 3 ⇒ 2AC = 5 × 3 ⇒ AC = 15 2
⇒ DC = AC – AD
Correct Option: D
As per the given in question , we draw a figure triangle ABC

Here , AE = 3 cm, AD = 2 cm and EB = 2 cm,
In ∆ ADE and ∆ ABC,
∠ABC = ∠ADE
∠A = ∠A
∴ ∆ ABC ~ ∆ ADE∴ AB = BC = AC AD DE AE ∴ AB + EB = AC AD AE ⇒ 3 + 2 = AC 2 3 ⇒ 5 = AC 2 3 ⇒ 2AC = 5 × 3 ⇒ AC = 15 2
⇒ DC = AC – ADDC = 15 - 2 = 15 - 4 = 11 2 2 2
DC = 5.5 cm
- ∆ ABC and ∆ DEF are similar. Also ∠A = ∠D and ∠B = ∠E. If 4AB = DE and BC = 12 cm, then EF is equal to
-
View Hint View Answer Discuss in Forum
As per the given in question , we draw figures of two similar triangles ABC and DEF
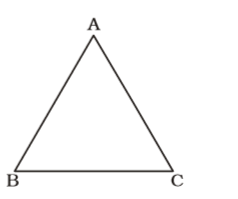
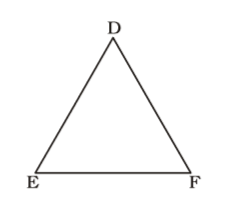
Given that , ∠A = ∠D and ∠B = ∠E and BC = 12 cm
∆ ABC ~ ∆ DEF and 4AB = DE⇒ AB = 1 DE 4
Correct Option: D
As per the given in question , we draw figures of two similar triangles ABC and DEF


Given that , ∠A = ∠D and ∠B = ∠E and BC = 12 cm
∆ ABC ~ ∆ DEF and 4AB = DE⇒ AB = 1 DE 4 ∴ AB = BC DE EF ⇒ 1 = 12 4 EF
⇒ EF = 48 cm.

