Plane Geometry
- O is the circumcentre of ∆ ABC. If ∠BAC = 85°, ∠BCA = 75°, then ∠OAC is equal to :
-
View Hint View Answer Discuss in Forum
According to question , we draw a figure of triangle ABC circumscribes a circle with centre O
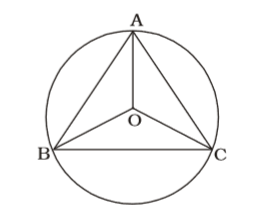
In ∆ ABC,
Given , ∠ BAC = 85° , ∠ BCA = 75°
∴ ∠ABC + ∠ BAC + ∠ BCA = 180°
∴ ∠ABC = 180° – 85° – 75° = 20°
We can say that the angle subtended by an arc of a circle at the centre is double the angle subtended by it at any point on the remaining part of the circle.
∴ ∠AOC = ∠ABC = 40°
∴ OA = OC = radii
In ∆ OAC, ∠OAC = ∠OCACorrect Option: B
According to question , we draw a figure of triangle ABC circumscribes a circle with centre O

In ∆ ABC,
Given , ∠ BAC = 85° , ∠ BCA = 75°
∴ ∠ABC + ∠ BAC + ∠ BCA = 180°
∴ ∠ABC = 180° – 85° – 75° = 20°
We can say that the angle subtended by an arc of a circle at the centre is double the angle subtended by it at any point on the remaining part of the circle.
∴ ∠AOC = ∠ABC = 40°
∴ OA = OC = radii
In ∆ OAC, ∠OAC = ∠OCA
(The angles at the base of an isosceles triangle are equal)
∠OAC + ∠OCA = 180° – 40° = 140°∴ ∠OAC = 140° = 70° 2
- ABC is a cyclic triangle and the bisectors of ∠BAC, ∠ABC and ∠BCA meet the circle at P, Q, and R respectively. Then the angle ∠RQP is
-
View Hint View Answer Discuss in Forum
As per the given in question , we draw a figure of a cyclic triangle ABC and the bisectors of ∠BAC, ∠ABC and ∠BCA meet the circle at P, Q, and R

∠BQP =∠BAP∴ ∠BQP = ∠A 2
∠BQR = ∠BCR∴ ∠BQR = 1 ∠C 2 ∴ ∠BQP + ∠BQR = 1 (∠A + ∠C) 2
Correct Option: A
As per the given in question , we draw a figure of a cyclic triangle ABC and the bisectors of ∠BAC, ∠ABC and ∠BCA meet the circle at P, Q, and R

∠BQP =∠BAP∴ ∠BQP = ∠A 2
∠BQR = ∠BCR∴ ∠BQR = 1 ∠C 2 ∴ ∠BQP + ∠BQR = 1 (∠A + ∠C) 2 ⇒ ∠PQR = 1 (180° - ∠B) 2
[we know that , ∠A + ∠B + ∠C = 180°]∠PQR = 90° – B 2
- The chord of a circle is equal to its radius. The angle subtended by this chord at the minor arc of the circle is
-
View Hint View Answer Discuss in Forum
According to question , we draw a figure of a circle with centre O

Here , OA = AB = OB
∠AOB = 60°
∴ ∠ADB = 30°
As we know that Angle subtended at the centre by an arc is twice of that subtended at the circumference.
☐ ADBC is a cyclic quadrilateral.Correct Option: C
According to question , we draw a figure of a circle with centre O

Here , OA = AB = OB
∠AOB = 60°
∴ ∠ADB = 30°
As we know that Angle subtended at the centre by an arc is twice of that subtended at the circumference.
☐ ADBC is a cyclic quadrilateral.
∴ ∠ADB + ∠ACB = 180°
⇒ 30° + ∠ACB = 180°
⇒ ∠ACB = 180° – 30° = 150°
- ‘O’ is the circumcentre of triangle ABC. If ∠ BAC = 50° then ∠ OBC is
-
View Hint View Answer Discuss in Forum
According to question , we draw a figure of triangle ABC whose O is the circumcentre

∠BAC = Angle at circumference = 50°
∠BOC = angle at centre = 2∠BAC = 100°
OB = OC = radiusCorrect Option: D
According to question , we draw a figure of triangle ABC whose O is the circumcentre

∠BAC = Angle at circumference = 50°
∠BOC = angle at centre = 2∠BAC = 100°
OB = OC = radius∴ ∠OBC = ∠OCB = 80° = 40° 2
- The radii of two concentric circles are 17 cm and 25 cm. A straight line PQRS intersects the larger circle at the points P and S and intersects the smaller circle at the points Q and R. If QR = 16 cm, then the length (in cm.) of PS is
-
View Hint View Answer Discuss in Forum
According to question , we draw a figure of two concentric circles whose radii are 17 cm and 25 cm

Point ‘O’ is the centre of circle. OD is perpendicular on QR.
Here , QD = DR = 8 cm.
From ∆ OQD,
OD = √OQ² - QD²
OD = √17² - 8²
OD = √(17 + 8)(17 - 8)
OD = √25 × 9 = 5 × 3 = 15 cm.
From ∆ OPD,
PD = √OP² - OD²Correct Option: D
According to question , we draw a figure of two concentric circles whose radii are 17 cm and 25 cm

Point ‘O’ is the centre of circle. OD is perpendicular on QR.
Here , QD = DR = 8 cm.
From ∆ OQD,
OD = √OQ² - QD²
OD = √17² - 8²
OD = √(17 + 8)(17 - 8)
OD = √25 × 9 = 5 × 3 = 15 cm.
From ∆ OPD,
PD = √OP² - OD²
PD = √25² - 15²
PD = √(25 + 15)(25 - 15)
PD = √40 × 10 = 20 cm.
∴ PS = 2PD = 2 × 20 = 40 cm.

