Plane Geometry
- Two supplementary angles are in the ratio 2 : 3. The angles are
-
View Hint View Answer Discuss in Forum
As per the given question ,
Ratio of supplementary angles = 2 : 3
Let the supplementary angles are 2y and 3y .
As we now that the sum of supplementary angles is 180°.
∴ 2y + 3y = 180Correct Option: C
As per the given question ,
Ratio of supplementary angles = 2 : 3
Let the supplementary angles are 2y and 3y .
As we now that the sum of supplementary angles is 180°.
∴ 2y + 3y = 180⇒ 5y = 180 ⇒ y = 180 = 36 5
∴ 2y = 2 × 36 = 72° and, 3y = 3 × 36 = 108°
- In ∆ABC ∠A = ∠B = 60°, AC = √13 cm. The lines AD and BD intersect at D with ∠D= 90°. If DB = 2 cm, then the length of AD is
-
View Hint View Answer Discuss in Forum
As per the given in question , we draw a figure of a triangle ABC

Here , ∠A = ∠B = ∠C = 60°
BC = AB = AC = √13 cm
BD = 2 cm
In ∆ ABD,
AB² = BD² + AD²Correct Option: A
As per the given in question , we draw a figure of a triangle ABC

Here , ∠A = ∠B = ∠C = 60°
BC = AB = AC = √13 cm
BD = 2 cm
In ∆ ABD,
AB² = BD² + AD²
⇒ (√13)² = (2)² + AD²
⇒ AD² = 13 – 4 = 9
⇒ AD = √9 = 3 cm
- D is a point on the side BC of a triangle ABC such that AD ⊥BC. E is a point on AD for which AE : ED = 5 : 1. If ∠BAD = 30° and tan (∠ ACB) = 6 tan (∠DBE), then ∠ACB =
-
View Hint View Answer Discuss in Forum
According to question , we draw a figure of a triangle ABC
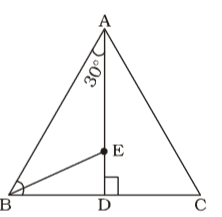
Given , ∠ BAD = 30° , AE : ED = 5 : 1 and tan (∠ ACB) = 6 tan (∠DBE)
∠ ABD = 60° [∵ ∠ADB = 90°]tan ∠ACB = AD/DC tan ∠DBE DE/BD = AD × BD = 6 BD DC DE DC
Correct Option: C
According to question , we draw a figure of a triangle ABC

Given , ∠ BAD = 30° , AE : ED = 5 : 1 and tan (∠ ACB) = 6 tan (∠DBE)
∠ ABD = 60° [∵ ∠ADB = 90°]tan ∠ACB = AD/DC tan ∠DBE DE/BD = AD × BD = 6 BD DC DE DC ∴ 6 BD = 6 DC
⇒ BD = DC
∆ ADB ≌ ∆ ADC
⇒ ∠ABD = ∠ACD = 60°
∴∠ ACB = 60°
Hence, ∆ ABC is an equilateral triangle
- All sides of a quadrilateral ABCD touch a circle. If AB = 6 cm, BC = 7.5 cm, CD = 3 cm, then DA is
-
View Hint View Answer Discuss in Forum
As per the given in question , we draw a figure of quadrilateral ABCD and its all sides touch a circle

Given , AB = 6 cm, BC = 7.5 cm, CD = 3 cm,
AE = AH
BE = BF
GC = FC
GD = HD
⇒ AE + BE + GC + GD = AH + BF + FC + HDCorrect Option: D
As per the given in question , we draw a figure of quadrilateral ABCD and its all sides touch a circle

Given , AB = 6 cm, BC = 7.5 cm, CD = 3 cm,
AE = AH
BE = BF
GC = FC
GD = HD
⇒ AE + BE + GC + GD = AH + BF + FC + HD
⇒ AB + CD = AD + BC
⇒ 6 + 3 = AD + 7.5
⇒ AD = 9 – 7.5 = 1.5 cm
- ∠A, ∠B, ∠C are three angles of a triangle. If ∠A – ∠B = 15°, ∠B – ∠C = 30°, then ∠A, ∠B and ∠C are
-
View Hint View Answer Discuss in Forum
Here , ∠B – ∠C = 30°, ∠A – ∠B = 15°
∴ ∠A + ∠B + ∠C = 180° ....(i)
(∠B – ∠C) – (∠A – ∠B) = 30° – 15°
⇒ 2 ∠B – ∠A – ∠C = 15° ....(ii)
By adding (i) and (ii), we get
3∠B = 180° + 15° = 195°
⇒ ∠B = 65°
∠A – ∠B = 15°Correct Option: C
Here , ∠B – ∠C = 30°, ∠A – ∠B = 15°
∴ ∠A + ∠B + ∠C = 180° ....(i)
(∠B – ∠C) – (∠A – ∠B) = 30° – 15°
⇒ 2 ∠B – ∠A – ∠C = 15° ....(ii)
By adding (i) and (ii), we get
3∠B = 180° + 15° = 195°
⇒ ∠B = 65°
∠A – ∠B = 15°
⇒ ∠A = 15° + 65° = 80°
And ∠B – ∠C = 30°
⇒ ∠C = ∠B – 30° = 65° – 30° = 35°

