Plane Geometry
- Let ABC be an equilateral triangle and AX, BY, CZ be the altitudes. Then the right statement out of the four given responses is
-
View Hint View Answer Discuss in Forum
According to question ,
In an equilateral ∆ABC,
We know that , ∠ A = ∠ B = ∠C = 60°
AB = BC = CACorrect Option: A
According to question ,
In an equilateral ∆ABC,
We know that , ∠ A = ∠ B = ∠C = 60°
AB = BC = CA
∴ AX = BY = CZ
- If the incentre of an equilateral triangle lies inside the triangle and its radius is 3 cm, then the side of the equilateral triangle is
-
View Hint View Answer Discuss in Forum
As we know that ,
In radius = Side 2√3
Correct Option: B
As we know that ,
In radius = Side 2√3 ⇒ 3 = Side ⇒ Side = 3 × 2√3 2√3
∴ Side = 6 √3 cm.
- If ABC is an equilateral triangle and P, Q, R respectively denote the middle points of AB, BC, CA then.
-
View Hint View Answer Discuss in Forum
The line segments joining the mid points of the sides of a triangle form four triangles, each of which is similar to the original triangle.
Correct Option: A
The line segments joining the mid points of the sides of a triangle form four triangles, each of which is similar to the original triangle. Hence PQR must be an equilateral triangle .
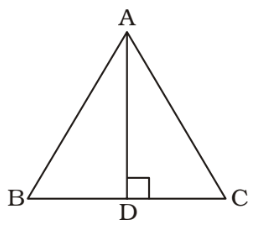
- If ABC is an equilateral triangle and D is a point on BC such that AD ⊥ BC, then
-
View Hint View Answer Discuss in Forum
According to given question, we draw a figure of an equilateral triangle ABC.
Correct Option: C
According to given question, we draw a figure of an equilateral triangle ABC.

Let us assume AB = 2Q units
⇒ BD = DC = Q units
∴ AB : BD = 2 : 1
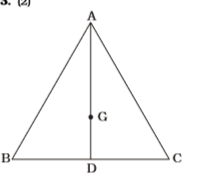
- G is the centroid of the equilateral triangle ABC. If AB = 10 cm then length of AG is
-
View Hint View Answer Discuss in Forum
First of all , We draw a figure of equilateral triangle ABC whose length is 10 cm ,
AB = 10 cm
BD = 5 cm
∠ ADB = 90°
∴ AD = √AB² - BD²
AD = √10² - 5²
AD = √100 - 25
AD = √75
AD = 5√3 cmCorrect Option: B
First of all , We draw a figure of equilateral triangle ABC whose length is 10 cm ,

AB = 10 cm
BD = 5 cm
∠ ADB = 90°
∴ AD = √AB² - BD²
AD = √10² - 5²
AD = √100 - 25
AD = √75
AD = 5√3 cmAG = 2 AD = 2 × 5 √3 3 3 AG = 10 √3 cm 3

