Plane Geometry
- In a triangle ABC, OB and OC are the bisectors of angles ∠B and ∠C respectively. ∠BAC = 60°. The angle ∠BOC will be :
-
View Hint View Answer Discuss in Forum
On the basis of question we draw a figure of triangle ABC in which OB and OC are the bisectors of angles ∠B and ∠C respectively ,
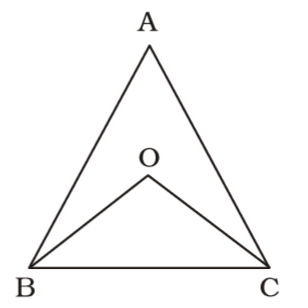
In ∆ABC,
Given , ∠BAC = 60°
∴ ∠ABC + ∠ACB = 180° – 60° = 120°⇒ 1 (∠ABC + ∠ACB) = 60° 2
In ∆BOC,
⇒ ∠OBC + ∠OCB + ∠BOC = 180°
Correct Option: B
On the basis of question we draw a figure of triangle ABC in which OB and OC are the bisectors of angles ∠B and ∠C respectively ,

In ∆ABC,
Given , ∠BAC = 60°
∴ ∠ABC + ∠ACB = 180° – 60° = 120°⇒ 1 (∠ABC + ∠ACB) = 60° 2
In ∆BOC,
⇒ ∠OBC + ∠OCB + ∠BOC = 180°⇒ 1 (∠ABC + ∠ACB) + ∠BOC = 180° 2
⇒ ∠BOC = 180° – 60° = 120°
- The point where the all three medians of a triangle meet is called
-
View Hint View Answer Discuss in Forum
The point of intersection of medians of a triangle is called centroid.
Correct Option: A
As we know that the point of intersection of medians of a triangle is called centroid.
- G and AD are respectively the centroid and median of the triangle ∆ABC.The ratio AG : AD is equal to
-
View Hint View Answer Discuss in Forum
On the basis of question we draw a figure of triangle ABC in which G and AD are respectively the centroid and median ,
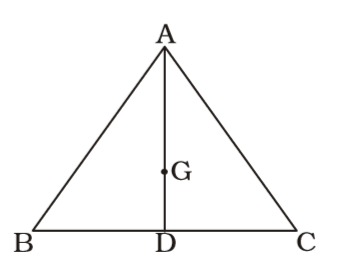
The point of intersection of medians of a triangle is called centroid. It divides each median in the ratio 2 : 1.∴ AG = 2 ⇒ GD = 1 GD 1 AG 2 ⇒ GD + 1 = 1 + 1 AG 2 ⇒ GD + AG = 1 + 2 AG 2
Correct Option: B
On the basis of question we draw a figure of triangle ABC in which G and AD are respectively the centroid and median ,

The point of intersection of medians of a triangle is called centroid. It divides each median in the ratio 2 : 1.∴ AG = 2 ⇒ GD = 1 GD 1 AG 2 ⇒ GD + 1 = 1 + 1 AG 2 ⇒ GD + AG = 1 + 2 AG 2 ⇒ AD = 3 AG 2
⇒ AG : AD = 2 : 3
- The side BC of ∆ ABC is extended to the point D. If ∠ACD = 112° and ∠B = 3/4 ∠A, then the value of ∠B is
-
View Hint View Answer Discuss in Forum
As per the given in question , we draw a figure triangle ABC whose side BC is extended to the point D
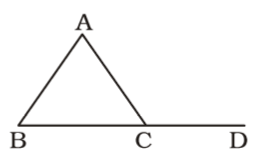
∵ Exterior angle of triangle is equal to the sum of two opposite angles.
∵ ∠ACD = 112°
∴ ∠A + ∠B = 112°⇒ 4 ∠B + ∠B = 112° 3 ⇒ 4∠B + 3∠B = 112° 3 
∵ ∠B = 3 ∠A ⇒ ∠A = 4 ∠B 
4 3
⇒ 7 × ∠B = 112 × 3
Correct Option: B
As per the given in question , we draw a figure triangle ABC whose side BC is extended to the point D

∵ Exterior angle of triangle is equal to the sum of two opposite angles.
∵ ∠ACD = 112°
∴ ∠A + ∠B = 112°⇒ 4 ∠B + ∠B = 112° 3 ⇒ 4∠B + 3∠B = 112° 3 
∵ ∠B = 3 ∠A ⇒ ∠A = 4 ∠B 
4 3
⇒ 7 × ∠B = 112 × 3⇒ ∠B = 112 × 3 = 48° 7
- If the angles of a triangle are in the ratio of 2 : 3 : 4, then the difference of the measure of greatest angle and smallest angle is
-
View Hint View Answer Discuss in Forum
We know that Sum of three angles of a triangle = 180°
A : B : C = 2 : 3 : 4
Sum of the terms of ratio = 2 + 3 + 4 = 9Required difference = 
4 - 2 
× 180° 9 9
Correct Option: C
We know that Sum of three angles of a triangle = 180°
A : B : C = 2 : 3 : 4
Sum of the terms of ratio = 2 + 3 + 4 = 9Required difference = 
4 - 2 
× 180° 9 9 Required difference = 2 × 180° = 40° 9

