Plane Geometry
- If I be the incentre of ∆ ABC and ∠B = 70° and ∠C = 50°, then the magnitude of ∠BIC is
-
View Hint View Answer Discuss in Forum
Firstly , We draw a figure of triangle ABC whose I is incentre ,
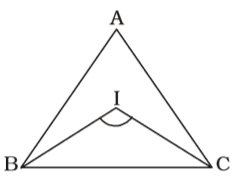
Here , ∠B = 70° and ∠C = 50°∠IBC = 70° = 35° ; 2 ∠ICB = 50° = 25° ; 2
Correct Option: C
Firstly , We draw a figure of triangle ABC whose I is incentre ,

Here , ∠B = 70° and ∠C = 50°∠IBC = 70° = 35° ; 2 ∠ICB = 50° = 25° ; 2
We know that , ∠BIC + ∠ICB + ∠IBC = 180°
∴ ∠BIC = 180° – 35° – 25°
∠BIC = 180° – 60° = 120°
- In a triangle ABC, AB + BC = 12 cm, BC + CA = 14 cm and CA + AB = 18 cm. Find the radius of the circle (in cm) which has the same perimeter as the triangle.
-
View Hint View Answer Discuss in Forum
Given that , AB + BC = 12
BC + CA = 14
CA + AB = 18
On adding , we get
∴ 2(AB + BC + CA) = 12 + 14 + 18 = 44
⇒ AB + BC + CA = 22
∴ 2πr = 22⇒ 2 × 22 × r = 22 7
Correct Option: B
Given that , AB + BC = 12
BC + CA = 14
CA + AB = 18
On adding , we get
∴ 2(AB + BC + CA) = 12 + 14 + 18 = 44
⇒ AB + BC + CA = 22
∴ 2πr = 22⇒ 2 × 22 × r = 22 7 ⇒ r = 7 cm 2
- In ∆ABC, PQ is parallel to BC. If AP : PB = 1 : 2 and AQ = 3 cm; AC is equal to
-
View Hint View Answer Discuss in Forum
Firstly , We draw a figure of triangle ABC ,
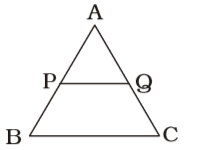
Here , AP : PB = 1 : 2AP = AQ = 1 PB QC 2
Correct Option: B
Firstly , We draw a figure of triangle ABC ,

Here , AP : PB = 1 : 2AP = AQ = 1 PB QC 2 ⇒ QC = 2 ⇒ QC + AQ = 3 AQ 1 AQ 1
⇒ AC = 3AQ = 9 cm
- I is the incentre of ∆ABC, ∠ABC = 60° and ∠ACB = 50°. Then ∠BIC is :
-
View Hint View Answer Discuss in Forum
On the basis of given in question , we draw a figure triangle ABC ,
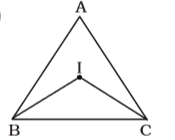
∠ABC = 60°, ACB = 50°∠IBC = 1 ∠ABC = 30° 2 ∠ICB = 1 ∠ACB = 25° 2
Correct Option: B
On the basis of given in question , we draw a figure triangle ABC ,

∠ABC = 60°, ACB = 50°∠IBC = 1 ∠ABC = 30° 2 ∠ICB = 1 ∠ACB = 25° 2
We know that , ∠BIC + ∠ICB + ∠IBC = 180°
∴ ∠BIC = 180° – 30° – 25° = 125°
- In an isosceles triangle, if the unequal angle is twice the sum of the equal angles, then each equal angle is
-
View Hint View Answer Discuss in Forum
We draw a figure of an isosceles triangle ABC ,
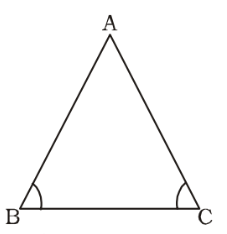
Given that , ∠ B = ∠ C
∴ ∠ A = 2(∠ B + ∠ C)
⇒ ∠ A = 4∠ C
we know that , ∠ A + ∠ B + ∠ C = 180°
∴ 4∠ C + ∠ C + ∠ C = 180°Correct Option: C
We draw a figure of an isosceles triangle ABC ,

Given that , ∠ B = ∠ C
∴ ∠ A = 2(∠ B + ∠ C)
⇒ ∠ A = 4∠ C
we know that , ∠ A + ∠ B + ∠ C = 180°
∴ 4∠ C + ∠ C + ∠ C = 180°
⇒ 6∠ C = 180°
⇒ ∠ C = 30°

