Plane Geometry
- In a ∆ABC, if 2 ∠ A = 3 ∠ B = 6∠C, value of ∠ B is
-
View Hint View Answer Discuss in Forum
As per the given in question,
∴ 2∠A = 3∠B = 6∠C⇒ 2∠A = 3∠B = 6∠C 6 6 6 ⇒ ∠A = ∠B = ∠C 3 2 1
⇒ ∠A : ∠B : ∠C = 3 : 2 : 1∴ ∠B = 
2 
× 180° 1 + 2 + 3
Correct Option: A
As per the given in question,
∴ 2∠A = 3∠B = 6∠C⇒ 2∠A = 3∠B = 6∠C 6 6 6 ⇒ ∠A = ∠B = ∠C 3 2 1
⇒ ∠A : ∠B : ∠C = 3 : 2 : 1∴ ∠B = 
2 
× 180° 1 + 2 + 3 ∠B = 2 × 180° = 60° 6
- If in a triangle ABC, D and E are on the sides AB and AC, such that, DE is parallel to BC and AD / BD = 3 / 5 . If AC = 4 cm, then AE is
-
View Hint View Answer Discuss in Forum
As per the given in question , we draw a figure of triangle ABC in which D and E are on the sides AB and AC, such that, DE is parallel to BC ,
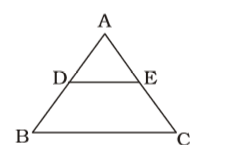
DE || BC ,∴ AD = AE AB AC AD = 3 ⇒ BD = 5 BD 5 AD 3 ⇒ BD + 1 = 5 + 1 AD 3 ⇒ BD + AD = 5 + 3 AD 3 ⇒ AB = 8 AD 3 ⇒ AD = 3 AB 8
Correct Option: A
As per the given in question , we draw a figure of triangle ABC in which D and E are on the sides AB and AC, such that, DE is parallel to BC ,

DE || BC ,∴ AD = AE AB AC AD = 3 ⇒ BD = 5 BD 5 AD 3 ⇒ BD + 1 = 5 + 1 AD 3 ⇒ BD + AD = 5 + 3 AD 3 ⇒ AB = 8 AD 3 ⇒ AD = 3 AB 8 ∴ AD = AE AB AC ⇒ 3 = AE ⇒ AE = 3 × 4 8 4 8
AE = 1.5 cm.
- AD is the median of a triangle ABC and O is the centroid such that AO = 10 cm. Length of OD (in cm) is
-
View Hint View Answer Discuss in Forum
On the basis of given in question , we draw a figure triangle ABC which AD is the median ,
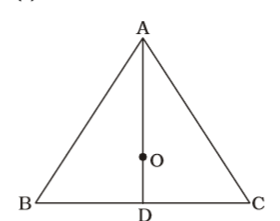
Point ‘O’ is centroid and AD is median.∴ OA = 2 AD 3 ⇒ 10 = 2 AD 3 ⇒ AD = 10 × 3 = 15 cm 2
Correct Option: C
On the basis of given in question , we draw a figure triangle ABC which AD is the median ,

Point ‘O’ is centroid and AD is median.∴ OA = 2 AD 3 ⇒ 10 = 2 AD 3 ⇒ AD = 10 × 3 = 15 cm 2 ∴ OD = 1 AD = 15 = 5 cm 3 3
- In a ∆ ABC, the medians AD, BE and CF meet at G, then which of the following is true?
-
View Hint View Answer Discuss in Forum
Firstly , We draw a figure of triangle ABC whose the medians AD, BE and CF meet at G ,

In any triangle the sum of the squares of any two sides is equal to twice the square of half of the third side together with twice the square of the median bisecting it.
∴ AB² + AC² = 2(AD² + BD²)
⇒ AB² + AC² = 2(AD² + BC²/4)
⇒ 2(AB² + AC²) = 4 AD² + BC²
Similarly,
2(AB² + BC²) = 4 BE² + AC²
2(AC² + BC²) = 4 CF² + AB²
On adding all three, we get
4(AB² + BC² + AC²) = 4(AD² + BE² + CF²) + BC² + AC² + AB²
⇒ 3(AB² + BC² + AC²) = 4(AD² + BE² + CF²)Correct Option: D
Firstly , We draw a figure of triangle ABC whose the medians AD, BE and CF meet at G ,

In any triangle the sum of the squares of any two sides is equal to twice the square of half of the third side together with twice the square of the median bisecting it.
∴ AB² + AC² = 2(AD² + BD²)
⇒ AB² + AC² = 2(AD² + BC²/4)
⇒ 2(AB² + AC²) = 4 AD² + BC²
Similarly,
2(AB² + BC²) = 4 BE² + AC²
2(AC² + BC²) = 4 CF² + AB²
On adding all three, we get
4(AB² + BC² + AC²) = 4(AD² + BE² + CF²) + BC² + AC² + AB²
⇒ 3(AB² + BC² + AC²) = 4(AD² + BE² + CF²)
Again,
AB + AC > 2AD
AB + BC > 2BE
BC + AC > 2CF
On adding , we get
∴ 2(AB + BC + AC) > 2(AD + BE + CF)
⇒ AB + BC + AC > AD + BE + CF
- In ∆ABC, D is the mid-point of BC. Length AD is 27 cm. N is a point in AD such that the length of DN is 12 cm. The distance of N from the centroid of ∆ ABC is equal to
-
View Hint View Answer Discuss in Forum
On the basis of given in question , we draw a figure triangle ABC ,
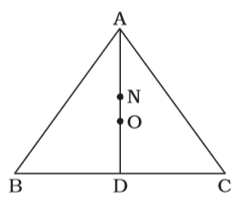
AD = 27 cm
Centroid = O
From figure we know that , AO : OD = 2 : 1∴ OD = 1 AD 3 OD = 1 × 27 = 9 cm 3
Correct Option: A
On the basis of given in question , we draw a figure triangle ABC ,

AD = 27 cm
Centroid = O
From figure we know that , AO : OD = 2 : 1∴ OD = 1 AD 3 OD = 1 × 27 = 9 cm 3
Given , ND = 12 cm
∴ ON = DN – OD = 12 – 9 = 3 cm

