Plane Geometry
- What is the position of the circumcentre of an obtuse–angled triangle?
-
View Hint View Answer Discuss in Forum
As per the given in question , we draw a figure obtuse–angled triangle ACD

Correct Option: B
As per the given in question , we draw a figure obtuse–angled triangle ACD

According to question, circumcentre lies outside the triangle.
- In &8710; ABC ∠BAC = 90° and AD ⊥ BC. If BD = 3 cm and CD = 4 cm, then the length of AD is
-
View Hint View Answer Discuss in Forum
Firstly , We draw a figure of triangle ABC

Given , BD = 3 cm
CD = 4 cm
In ∆ ABC,
AB² + AC² = 7²
⇒ AB² + AC² = 49 ....(i)
In ∆ ABD,
AB² = AD² + 3²
AB² = AD² + 9 ....(ii)
In ∆ ADC,
AC² = AD² + 16 ....(iii)
On adding equations (ii) and (iii),
AB² + AC² = AD² + 9 + AD² + 16
⇒ 49 = 2AD² + 25Correct Option: C
Firstly , We draw a figure of triangle ABC

Given , BD = 3 cm
CD = 4 cm
In ∆ ABC,
AB² + AC² = 7²
⇒ AB² + AC² = 49 ....(i)
In ∆ ABD,
AB² = AD² + 3²
AB² = AD² + 9 ....(ii)
In ∆ ADC,
AC² = AD² + 16 ....(iii)
On adding equations (ii) and (iii),
AB² + AC² = AD² + 9 + AD² + 16
⇒ 49 = 2AD² + 25
⇒ 2AD² = 49 – 25 = 24
⇒ AD² = 12
⇒ AD = √12 = 2√3 cm.
- The internal bisectors of the ∠B and ∠C of the ∆ ABC, intersect at O. If ∠A = 100°, then the measure of ∠BOC is :
-
View Hint View Answer Discuss in Forum
On the basis of given in question , we draw a figure triangle ABC ,
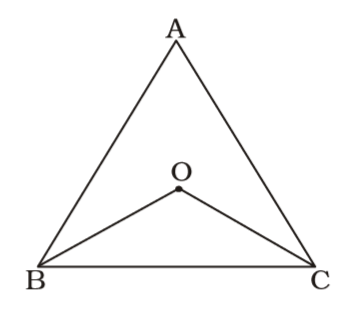
∠OBC = 1 ∠ABC; 2 ∠OCB = 1 ∠ACB; 2
From ∆ OBC,
∠OBC + ∠OCB + ∠BOC = 180°1 (∠ABC + ∠ACB) + ∠BOC = 180° 2 ⇒ 1 (180° - ∠BAC) + ∠BOC = 180° 2
Correct Option: A
On the basis of given in question , we draw a figure triangle ABC ,

∠OBC = 1 ∠ABC; 2 ∠OCB = 1 ∠ACB; 2
From ∆ OBC,
∠OBC + ∠OCB + ∠BOC = 180°1 (∠ABC + ∠ACB) + ∠BOC = 180° 2 ⇒ 1 (180° - ∠BAC) + ∠BOC = 180° 2 ⇒ 1 (180° - 100) + ∠BOC = 180° 2
⇒ ∠BOC = 180° – 40° = 140°
- G is the centroid of ∆ ABC. The medians AD and BE intersect at right angles. If the lengths of AD and BE are 9 cm and 12 cm respectively; then the length of AB (in cm) is
-
View Hint View Answer Discuss in Forum
Firstly , We draw a figure of triangle ABC whose G is the centroid ,
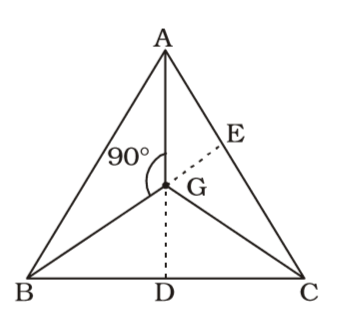
AD = 9 cm.∴ AG = 2 × 9 = 6 cm 3
BE = 12 cm.∴ BG = 2 × 12 = 8 cm 3
∠AGB = 90°
∴ From ∆ ABG,
AB = √AG² + BG²
AB= √6² + 8²
Correct Option: B
Firstly , We draw a figure of triangle ABC whose G is the centroid ,

AD = 9 cm.∴ AG = 2 × 9 = 6 cm 3
BE = 12 cm.∴ BG = 2 × 12 = 8 cm 3
∠AGB = 90°
∴ From ∆ ABG,
AB = √AG² + BG²
AB= √6² + 8²
AB = √36 + 64
AB = √100 = 10 cm.
- Internal bisectors of ∠Q and ∠R of ∆PQR intersect at O. If ∠ROQ = 96° then the vlaue of ∠RPQ is
-
View Hint View Answer Discuss in Forum
As per the given in question , we draw a figure of triangle ABC
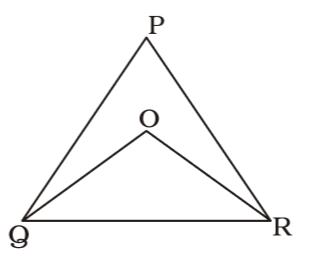
∠ROQ = 96°
In ∆ OQR
∠OQR + ∠ORQ + ∠QOR = 180°⇒ 1 ∠PQR + 1 ∠PRQ + 96° = 180° 2 2 ⇒ 1 (∠PQR + ∠PRQ) = 180° – 96° = 84° 2
Correct Option: C
As per the given in question , we draw a figure of triangle ABC

∠ROQ = 96°
In ∆ OQR
∠OQR + ∠ORQ + ∠QOR = 180°⇒ 1 ∠PQR + 1 ∠PRQ + 96° = 180° 2 2 ⇒ 1 (∠PQR + ∠PRQ) = 180° – 96° = 84° 2
⇒ ∠PQR + ∠PRQ = 2 × 84° = 168°
In ∆ PQR,
∴ ∠QPR = 180° – ( ∠PQR + ∠PRQ ) = 180° – 168° = 12°

