Plane Geometry
- In a triangle ABC, ∠BAC = 90° and AD is perpendicular to BC. If AD = 6 cm and BD = 4 cm, then the length of BC is
-
View Hint View Answer Discuss in Forum
On the basis of question we draw a figure of triangle ABC ,

∠BAC = 90°
AB = √AD² + BD²
AB = √6² + 4²
AB = √36 + 16 = √52 cm
∆ABD and ∆ABC are similar.Correct Option: D
On the basis of question we draw a figure of triangle ABC ,

∠BAC = 90°
AB = √AD² + BD²
AB = √6² + 4²
AB = √36 + 16 = √52 cm
∆ABD and ∆ABC are similar.∴ AB = BD BC AB
⇒ AB² = BC × BD
⇒ 52 = BC × 4
⇒ BC = 52 ÷ 4 = 13 cm
-
In ∆ ABC, ∠BAC = 90° and AB = 1 BC.Then the measure of ∠ACB is : 2
-
View Hint View Answer Discuss in Forum
On the basis of question we draw a figure of triangle ABC ,

Let AB = k ; BC = 2k units
⇒ AC = √4k² - k² = √3k
Correct Option: B
On the basis of question we draw a figure of triangle ABC ,

Let AB = k ; BC = 2k units
⇒ AC = √4k² - k² = √3k∴ ∠ACB = AB = 1 = sin30° BC 2
∴ ∠ACB = 30°
-
The ratio of the angles of a triangle is 1 : 2 : 3 .Then the smallest angle is : 3
-
View Hint View Answer Discuss in Forum
As per the given in question,
In a ∆ ABC,∠A : ∠B : ∠C = 1 : 2 : 3 = 3 : 2 : 9 3
Sum of the terms of ratio = 3 + 2 + 9 = 14∴ Lowest angle = ∠B = 2 × 180° 14
Correct Option: C
As per the given in question,
In a ∆ ABC,∠A : ∠B : ∠C = 1 : 2 : 3 = 3 : 2 : 9 3
Sum of the terms of ratio = 3 + 2 + 9 = 14∴ Lowest angle = ∠B = 2 × 180° 14 Lowest angle = ∠B = 180° = 25 5° 7 7
- In a triangle, the distance of the centroid from the three vertices is 4 cm, 6 cm and 8 cm respectively. Then the length of the smallest median is :
-
View Hint View Answer Discuss in Forum
On the basis of question we draw a figure of triangle ABC and the distance of the centroid from the three vertices is 4 cm, 6 cm and 8 cm respectively,
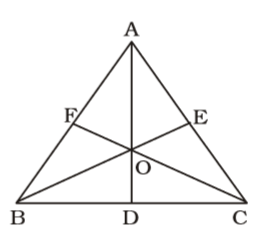
AO = 4 cm.⇒ 2 AD = 4 3 ⇒ AD = 4 × 3 = 6 cm. 2
BO = 6 cm.⇒ BE = 3 × 6 = 9 cm. 2
CO = 8 cm.
Correct Option: C
On the basis of question we draw a figure of triangle ABC and the distance of the centroid from the three vertices is 4 cm, 6 cm and 8 cm respectively,

AO = 4 cm.⇒ 2 AD = 4 3 ⇒ AD = 4 × 3 = 6 cm. 2
BO = 6 cm.⇒ BE = 3 × 6 = 9 cm. 2
CO = 8 cm.⇒ CF = 8 × 3 = 12 cm. 2
- The point of intersection of all the three medians of a triangle is called its
-
View Hint View Answer Discuss in Forum
We can say that the point of intersection of the medians of a triangle is called centroid.
Correct Option: C
We can say that the point of intersection of the medians of a triangle is called centroid. Hence , required answer is centroid .

