Plane Geometry
- Internal bisectors of ∠B and ∠C of ABC intersect at O. If ∠BOC = 102°, then the value of ∠BAC is
-
View Hint View Answer Discuss in Forum
As per the given in question , we draw a figure of a triangle ABC and the internal bisectors of ∠B and ∠C intersect at O
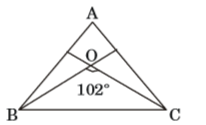
Here , ∠BOC = 102°
In ∆ABC ,
∠A + ∠B + ∠C = 180°⇒ ∠B + ∠C = 90° - ∠A .........( 1 ) 2 2 2
In ∆ BOC,∠BOC + ∠B + ∠C = 180° 2 2
Correct Option: B
As per the given in question , we draw a figure of a triangle ABC and the internal bisectors of ∠B and ∠C intersect at O

Here , ∠BOC = 102°
In ∆ABC ,
∠A + ∠B + ∠C = 180°⇒ ∠B + ∠C = 90° - ∠A .........( 1 ) 2 2 2
In ∆ BOC,∠BOC + ∠B + ∠C = 180° 2 2 ⇒ 102° + 90° – ∠A = 180° { ∴ Using ( 1 ) } 2 ⇒ ∠A = 102° + 90° - 180° = 12° 2
∴ ∠A = 24°
- A circle (with centre at O) is touching two intersecting lines AX and BY. The two points of contact A and B subtend an angle of 65° at any point C on the circumference of the circle. If P is the point of intersection of the two lines, then the measure of ∠APO is
-
View Hint View Answer Discuss in Forum
As per the given in question , we draw a figure of a circle with centre O

Given , ∠ACB = 65°
We can say that angle subtended at the centre of a circled by an arc is twice to that at the circumference.
∠AOB = 2 × ∠ACB = 2 × 65° = 130°Correct Option: A
As per the given in question , we draw a figure of a circle with centre O

Given , ∠ACB = 65°
We can say that angle subtended at the centre of a circled by an arc is twice to that at the circumference.
∠AOB = 2 × ∠ACB = 2 × 65° = 130°
∠OAP = 90°, ∠AOP = 65°
∴ ∠APO = 180° – 90° – 65° = 25°
- In ∆ABC, ∠B = 60° and ∠C = 40°. If AD and AE be respectively the internal bisector of ∠A and perpendicular on BC, then the measure of ∠DAE is
-
View Hint View Answer Discuss in Forum
According to question , we draw a figure of a
∆ABC in which AD and AE be respectively the internal bisector of ∠A and perpendicular on BC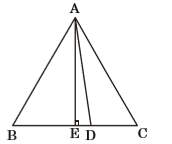
Given that , ∠B = 60° and ∠C = 40°
In ∆ABC ,
we know that , ∠A + ∠B + ∠C = 180°
∠A = 180° – 60° – 40° = 80°
∠ BAD = 80° ÷ 2 = 40°Correct Option: B
According to question , we draw a figure of a
∆ABC in which AD and AE be respectively the internal bisector of ∠A and perpendicular on BC
Given that , ∠B = 60° and ∠C = 40°
In ∆ABC ,
we know that , ∠A + ∠B + ∠C = 180°
∠A = 180° – 60° – 40° = 80°
∠ BAD = 80° ÷ 2 = 40°
∠BAE = 180° – 60° – 90° = 30°
∴ ∠DAE = ∠ BAD - ∠BAE
∴ ∠DAE = 40° – 30° = 10°
- The internal bisectors of ∠ABC and ∠ACB of ∆ABC meet each other at O. If ∠BOC =110°, then ∠BAC is equal to
-
View Hint View Answer Discuss in Forum
On the basis of question we draw a figure of a ∆ABC in which the internal bisectors of ∠ABC and ∠ACB meet each other at O ,

In ∆ ABC,
∠A + ∠B + ∠C = 180° ... (i)
In ∆ OBC,
We have , ∠OBC + ∠BOC + ∠OCB = 180°⇒ ∠B + 110° + ∠C = 180° 2 2
Correct Option: A
On the basis of question we draw a figure of a ∆ABC in which the internal bisectors of ∠ABC and ∠ACB meet each other at O ,

In ∆ ABC,
∠A + ∠B + ∠C = 180° ... (i)
In ∆ OBC,
We have , ∠OBC + ∠BOC + ∠OCB = 180°⇒ ∠B + 110° + ∠C = 180° 2 2 ⇒ ∠B + ∠C = 180° - 110° = 70° 2
⇒ ∠B + ∠C = 140°
From (i) , we get
∴ ∠A = 180° – 140° = 40°
- If ∆ ABC is similar to ∆DEF, such that ∠A = 47° and ∠E = 63° then ∠C is equal to :
-
View Hint View Answer Discuss in Forum
According to question , we draw a figure of two similar triangle ABC and DEF

∆ ABC ~ ∆ DEF
∴ ∠A = 47° = ∠D
∠B = ∠E = 63°Correct Option: B
According to question , we draw a figure of two similar triangle ABC and DEF

∆ ABC ~ ∆ DEF
∴ ∠A = 47° = ∠D
∠B = ∠E = 63°
In triangle ,
As we know that , ∠A + ∠B + ∠C = 180°
∴ ∠C = 180° – 47° – 63° =70°

