Internal bisectors of ∠B and ∠C of ABC intersect

Home » Aptitude » Plane Geometry » Question

Internal bisectors of ∠B and ∠C of ABC intersect at O. If ∠BOC = 102°, then the value of ∠BAC is

1. 12°
2. 24°
3. 48°
4. 60°

Correct Option: B

As per the given in question , we draw a figure of a triangle ABC and the internal bisectors of ∠B and ∠C intersect at O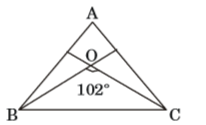

Here , ∠BOC = 102°
In ∆ABC ,
∠A + ∠B + ∠C = 180°

⇒	∠B	+	∠C	= 90° -	∠A	.........( 1 )
	2		2		2

In ∆ BOC,

∠BOC +	∠B	+	∠C	= 180°
	2		2

⇒ 102° + 90° –	∠A	= 180° { ∴ Using ( 1 ) }
	2

⇒	∠A	= 102° + 90° - 180° = 12°
	2

∴ ∠A = 24°