-
In ∆ABC, ∠B = 60° and ∠C = 40°. If AD and AE be respectively the internal bisector of ∠A and perpendicular on BC, then the measure of ∠DAE is
-
- 5°
- 10°
- 40°
- 60°
- 5°
Correct Option: B
According to question , we draw a figure of a
∆ABC in which AD and AE be respectively the internal bisector of ∠A and perpendicular on BC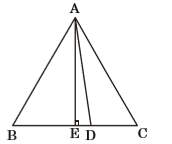
Given that , ∠B = 60° and ∠C = 40°
In ∆ABC ,
we know that , ∠A + ∠B + ∠C = 180°
∠A = 180° – 60° – 40° = 80°
∠ BAD = 80° ÷ 2 = 40°
∠BAE = 180° – 60° – 90° = 30°
∴ ∠DAE = ∠ BAD - ∠BAE
∴ ∠DAE = 40° – 30° = 10°

