According to question , we draw a figure of ∆ ABC in which inscribed in a circle

The angle at the circumference of a semi–circle is a right angle.
∴ ∠BAC = 90°
∠ABC = 36°
∴ ∠ACB = 90° – 36° = 54°
∠BCD = 90°

Correct Option: D

According to question , we draw a figure of ∆ ABC in which inscribed in a circle

The angle at the circumference of a semi–circle is a right angle.
∴ ∠BAC = 90°
∠ABC = 36°
∴ ∠ACB = 90° – 36° = 54°
∠BCD = 90°
∴ ∠ACD = 90° – 54° = 36°
∠DAC = 90°
∴ ∠ADC = 90° – 36° = 54°

As per the given in question , we draw a figure of two equal circles
Transverse common tangent = √d² - (r₁ + r₂)²
Transverse common tangent = √10² - (3 + 3)²
[ r₁ = r₂ = 3 cm.]
Transverse common tangent = √100 - 36

Correct Option: D

As per the given in question , we draw a figure of two equal circles
Transverse common tangent = √d² - (r₁ + r₂)²
Transverse common tangent = √10² - (3 + 3)²
[ r₁ = r₂ = 3 cm.]
Transverse common tangent = √100 - 36
Transverse common tangent = √64 = 8 cm.

According to question , we draw a figure

Correct Option: C

According to question , we draw a figure

Maximum No. of Common tangents = 4

As per the given in question , we draw a figure two circles touching each other externally

Correct Option: D

As per the given in question , we draw a figure two circles touching each other externally

No. of common tangents = 3

According to question , we draw a figure of a circle with centre O

OB = OC = radii
∴ In ∆ OBC,
OB = BC = CO

Correct Option: A

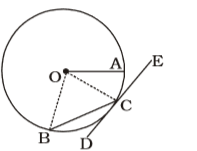
According to question , we draw a figure of a circle with centre O

OB = OC = radii
∴ In ∆ OBC,
OB = BC = CO
∴ ∠OCB = ∠OBC = ∠BOC = 60°
OC ⊥ DE
∴ ∠BCD = 90° – 60° = 30°