Electric circuits miscellaneous Easy Questions and Answers | Page - 15

Electric circuits miscellaneous

Consider the following circuit :

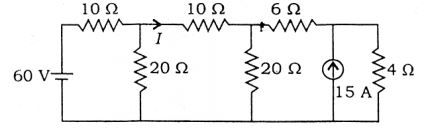
What is the current I in the above circuit?

1. 0 A
1. 2 A
1. 5 A
1. 6 A

View Hint View Answer Discuss in Forum

Using Thevenin’s theorem, we have

Since the two voltages are equal and in opposition, therefore I = 0.

Correct Option: A

Using Thevenin’s theorem, we have

Since the two voltages are equal and in opposition, therefore I = 0.

In the network shown in the given figure, if the voltage V at the time considered is 20 V, then
dV at that time will be
dt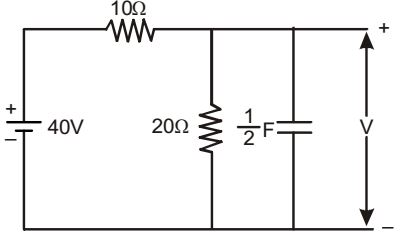

1. 1 V/s
1. 2 V/s
1. – 2 V/s
1. zero

View Hint View Answer Discuss in Forum

Given: V = 20 V
∴ Current through 20 ohm resistance = 20 = 1 A
20

Voltage across 10 ohm resistor = 40 – 20 = 20 V
Then current through the capacitor = 2 – 1 = 1 A.
∴ C dV = 1 dV A
dt 2 dt

⇒ dV = 2 V / s.
dt

Correct Option: B

Given: V = 20 V
∴ Current through 20 ohm resistance = 20 = 1 A
20

Voltage across 10 ohm resistor = 40 – 20 = 20 V
Then current through the capacitor = 2 – 1 = 1 A.
∴ C dV = 1 dV A
dt 2 dt

⇒ dV = 2 V / s.
dt

After closing the switch‘S’ at t = 0, the current i (t) at any instant ‘t’ in the network shown in the given figure will be

1. 10 + 10 e^{100 t}
1. 10 – 10 e^{100 t}
1. 10 + 10 e^{–100 t}
1. 10 – 10 e^{–100 t}

View Hint View Answer Discuss in Forum

It can be certified by checking the value of the current at t = 0₊ , and at t = ∞ which should be zero and 10/1 = 10 A respectively.
It is an R-L circuit
∴ i(t) = V_dc [1 - e^{(– R / L)t]}
R

Correct Option: D

It can be certified by checking the value of the current at t = 0₊ , and at t = ∞ which should be zero and 10/1 = 10 A respectively.
It is an R-L circuit
∴ i(t) = V_dc [1 - e^{(– R / L)t]}
R

The circuit shown in the given figure is steady-state with switch ‘S’ open. The switch is closed at t = 0. The values of v_c (0⁺) and v_c (∞) will be respectively

1. 2 V, 0 V
1. 0 V, 2 V
1. 2 V, 2 V
1. 0 V, 0 V

View Hint View Answer Discuss in Forum

When switchs had been open, the current of 2 A flows through R₂ alone. Thus voltage 2 V, and since there is no current in branch 1, the voltage acoss the capacitor is also 2V.
∴ v_c (0) = 2
Since after the switching action, voltage across the capacitor cannot change instantaneously,
∴ v_c (∞) = v_c (0) = 2V.
After the switch is closed and steady state condition reached, the entire current of 2A passes through the closed switch and voltage across the capacitor becomes zero.

Correct Option: A

When switchs had been open, the current of 2 A flows through R₂ alone. Thus voltage 2 V, and since there is no current in branch 1, the voltage acoss the capacitor is also 2V.
∴ v_c (0) = 2
Since after the switching action, voltage across the capacitor cannot change instantaneously,
∴ v_c (∞) = v_c (0) = 2V.
After the switch is closed and steady state condition reached, the entire current of 2A passes through the closed switch and voltage across the capacitor becomes zero.

In the circuit shown in the given, the switch S is open for a long time and closed at t = 0.

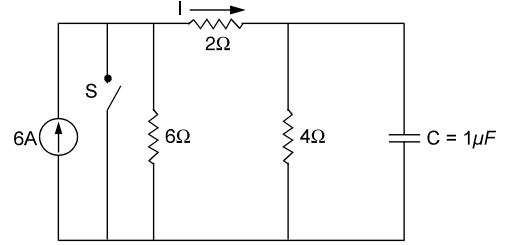
The value I at t = 0⁺ is

1. – 6A
1. – 3/2 A
1. 3 A
1. 9/2 A

View Hint View Answer Discuss in Forum

Voltage across capacitor prior to closing of switch
= 6 × 4 = 12 V
2

Immediately after closing of the switch S, the source current is all diverted into the ‘short’
and capacitor furnishes a current of 12 = 6 A in the 2-ohm resistor.
2

The direction is opposite to the marked one.
∴ I = – 6A.

Correct Option: A

Voltage across capacitor prior to closing of switch
= 6 × 4 = 12 V
2

Immediately after closing of the switch S, the source current is all diverted into the ‘short’
and capacitor furnishes a current of 12 = 6 A in the 2-ohm resistor.
2

The direction is opposite to the marked one.
∴ I = – 6A.