Electric circuits miscellaneous
- Consider a one-turn rectangular loop of wire place in a uniform magnetic field as shown in the figure. The plane of the loop is perpendicular to the field lines. The resistance of the loop is 0.4 Ω, and its inductance is negligible. The magnetic flux density (in Tesla) is a function of time, and is given by B(t) = 0.25 sinωt, where ω = 2π × 50 radian/second. The power absorbed (in Watt) by the loop from the magnetic field is __________.
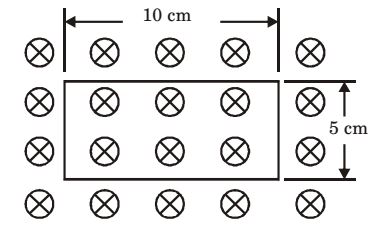
-
View Hint View Answer Discuss in Forum
P = V2emf R Vemf = -dΨ dt 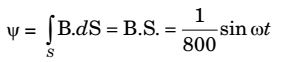
Vemf = -dΨ = -1 π cos ωt dt 8 P = π2 cos2 ωt × 1 64 R P = π2 
1 + cos 2ωt 
0.4 × 64 2 Pavg = π2 + π2 cos2ωt 20 × 0.4 × 64 0.4 × 64 × 2 Pavg = π2 = 0.192 W 20 × 0.4 × 64 Correct Option: A
P = V2emf R Vemf = -dΨ dt 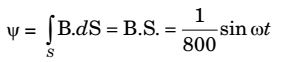
Vemf = -dΨ = -1 π cos ωt dt 8 P = π2 cos2 ωt × 1 64 R P = π2 
1 + cos 2ωt 
0.4 × 64 2 Pavg = π2 + π2 cos2ωt 20 × 0.4 × 64 0.4 × 64 × 2 Pavg = π2 = 0.192 W 20 × 0.4 × 64
- For a given circuit the thevenin equivalent is to be determined. The thevenin voltage VTh(in volts), seen from terminal AB is __________.
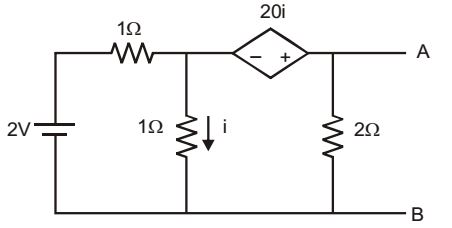
-
View Hint View Answer Discuss in Forum

Apply KVL
2 = 1(i + I1 ) + 1i
2 = 2i + I1 .......(i)
2 = 1(i + I1 ) - 20i + 2I1
2 = – 19i + 3I1 .......(ii)
Solving equations, (i) & (ii)
I1 = 1.68 Amp
Thevenin’s voltage,
Vth = 2I1 volt = 2 × 1.68
Vth = 3.36 voltCorrect Option: C

Apply KVL
2 = 1(i + I1 ) + 1i
2 = 2i + I1 .......(i)
2 = 1(i + I1 ) - 20i + 2I1
2 = – 19i + 3I1 .......(ii)
Solving equations, (i) & (ii)
I1 = 1.68 Amp
Thevenin’s voltage,
Vth = 2I1 volt = 2 × 1.68
Vth = 3.36 volt
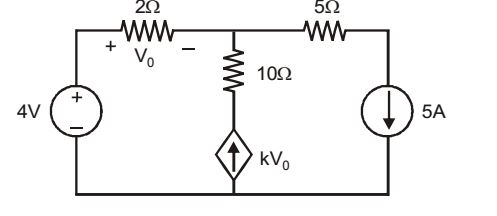
- In the given circuit parameter k is positive and power dissipated in 2 Ω resistor is 12.5W. The value of k is __________.
-
View Hint View Answer Discuss in Forum

Power dissipated in 2Ω P = I2R
12.5 = I2 × 2
I = 2.5 Amp
V0 = I × 2 = 2.5 × 2
V0 = 5 volt
Apply KCL,
I + KV0 = 5
2.5 + K × 5 = 5K = 0.5 Correct Option: D

Power dissipated in 2Ω P = I2R
12.5 = I2 × 2
I = 2.5 Amp
V0 = I × 2 = 2.5 × 2
V0 = 5 volt
Apply KCL,
I + KV0 = 5
2.5 + K × 5 = 5K = 0.5
- In a linear two-port network, when 10 V is applied to Port 1, a current of 4 A flows through Port 2 when it is short-circuited. When 5V is applied to Port 1, a current of 1.25 A flows through a 1Ω resistance connected across Port 2. When 3V is applied to Port 1, then current (in Ampere) through a 2 Ω resistance connect ed acoss Port 2 is _________.
-
View Hint View Answer Discuss in Forum
Case (i) :

We know that, I2 = Y21V1 + Y22 V2
4 = Y21 × 10 + Y22 × 0Y21 = 4 = 0.4 ℧ 10
Case (ii) :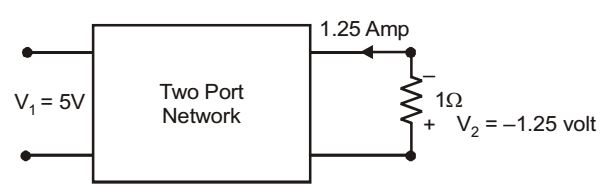
I2 = Y21V1 + Y22V2
1.25 = 0.4 × 5 + Y22 (– 1.25)
– 0.75 = – 1.25 Y22Y22 = 0.6 ℧
Case (iii) :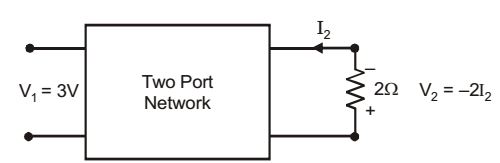
I2 = Y21V1 + Y22V2
I2 = 0.4(3) + 0.6 (– 2I2)
I2 = 1.2 – 1.2I2
2.2 I2 = 1.2I2 = 1.2 2.2 I2 = 0.545 Amp Correct Option: A
Case (i) :

We know that, I2 = Y21V1 + Y22 V2
4 = Y21 × 10 + Y22 × 0Y21 = 4 = 0.4 ℧ 10
Case (ii) :
I2 = Y21V1 + Y22V2
1.25 = 0.4 × 5 + Y22 (– 1.25)
– 0.75 = – 1.25 Y22Y22 = 0.6 ℧
Case (iii) :
I2 = Y21V1 + Y22V2
I2 = 0.4(3) + 0.6 (– 2I2)
I2 = 1.2 – 1.2I2
2.2 I2 = 1.2I2 = 1.2 2.2 I2 = 0.545 Amp
- A parallel plate capacitor is partially filled with glass of dielectric constant 4.0 as shown below. The dielectric strengths of air and glass are 30 kV/cm and 300 kV/cm, respectively. The maximum voltage (in kilovolts), which can be applied across the capacitor without any breakdown, is _______.
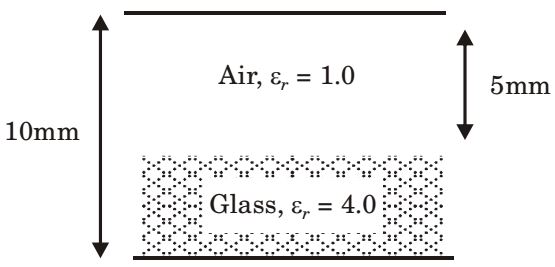
-
View Hint View Answer Discuss in Forum
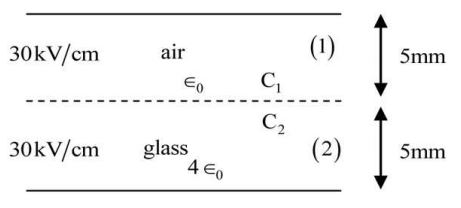
C1 = A∈0 d C2 = 4A∈0 d Ceq = C1 C2 = 4A∈0 C1 + C2 5d Dn = ρs = Q = CV = Ceqv A A A Dn = 4A∈0 V 5dA Dn = 
4 ∈0 
V 5dA E1 = Dn = 4 V ∈0 5d 30 × 105 = 4V 4d ⇒ V = 30 × 5 × 5 × 10-3 × 105 = 18.75 kV 4
Correct Option: A

C1 = A∈0 d C2 = 4A∈0 d Ceq = C1 C2 = 4A∈0 C1 + C2 5d Dn = ρs = Q = CV = Ceqv A A A Dn = 4A∈0 V 5dA Dn = 
4 ∈0 
V 5dA E1 = Dn = 4 V ∈0 5d 30 × 105 = 4V 4d ⇒ V = 30 × 5 × 5 × 10-3 × 105 = 18.75 kV 4

