Profit and Loss
- A person buys some pencils at 5 for a rupee and sells them at 3 for a rupee. His gain percent wll be :
-
View Hint View Answer Discuss in Forum
Given that , C.P. of 5 pencils = $ 1.
S.P. of 5 pencils = $ 5 3 Gain = 5 - 1 = 2 3 3 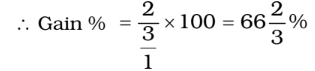
We can find required answer with the help of given formula :
Here, a = 5, p = 1 and b = 3, q = 1Gain % = 
aq - bp × 100% 
bp
Correct Option: A
Given that , C.P. of 5 pencils = $ 1.
S.P. of 5 pencils = $ 5 3 Gain = 5 - 1 = 2 3 3 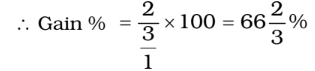
We can find required answer with the help of given formula :
Here, a = 5, p = 1 and b = 3, q = 1Gain % = 
aq - bp × 100% 
bp Gain % = 5 - 3 × 100% 3 Gain % = 200 = 66 2 % 3 3
- 100 oranges are bought for $ 350 and sold at the rate of 48 per dozen. The percentage of profit or loss is :
-
View Hint View Answer Discuss in Forum
Given in question , C.P. of 100 oranges = $ 350
S.P. of 12 oranges = $ 48∴ S.P. of 100 oranges = 48 × 100 = $ 400 12
Profit = S.P. - C.P. = $ (400 – 350) = $ 50∴ profit % = 50 × 100 = 100 % 350 7 profit % = 14 2 % 7
We can find required answer with the help of given formula :
Here, a = 100, p = 350b = 100, q = 48 × 100 = 400 12 Gain % = 
aq - bp 
× 100% bp
Correct Option: D
Given in question , C.P. of 100 oranges = $ 350
S.P. of 12 oranges = $ 48∴ S.P. of 100 oranges = 48 × 100 = $ 400 12
Profit = S.P. - C.P. = $ (400 – 350) = $ 50∴ profit % = 50 × 100 = 100 % 350 7 profit % = 14 2 % 7
We can find required answer with the help of given formula :
Here, a = 100, p = 350b = 100, q = 48 × 100 = 400 12 Gain % = 
aq - bp 
× 100% bp Gain % = 100 × 400 - 100 × 350 × 100% 100 × 350 Gain % = 40 - 35 × 100% 35 Gain % = 100 % = 14 2 % 7 7
- Oranges are bought at the rate of 10 for $ 25 and sold at the rate of 9 for $ 25. The profit percent is
-
View Hint View Answer Discuss in Forum
Suppose the number of oranges bought = LCM of 10 and 9 = 90
C.P. of 90 oranges = 25 × 90 = $225 10 S.P. of 90 oranges = 25 × 90 = $ 250 9
Profit = S.P. of 90 oranges - C.P. of 90 oranges = 250 - 225 = $ 25Profit % = 25 × 100 225 Profit % = 100 = 11 1 % 9 9
Second method to solve this question :
Here, a = 10, p = 25 and b = 9, q = 25Gain % = 
aq - bp 
× 100% bp Gain % = 
10 × 25 - 9 × 25 
× 100% 9 × 25
Correct Option: C
Suppose the number of oranges bought = LCM of 10 and 9 = 90
C.P. of 90 oranges = 25 × 90 = $225 10 S.P. of 90 oranges = 25 × 90 = $ 250 9
Profit = S.P. of 90 oranges - C.P. of 90 oranges = 250 - 225 = $ 25Profit % = 25 × 100 225 Profit % = 100 = 11 1 % 9 9
Second method to solve this question :
Here, a = 10, p = 25 and b = 9, q = 25Gain % = 
aq - bp 
× 100% bp Gain % = 
10 × 25 - 9 × 25 
× 100% 9 × 25 Gain % = 
250 - 225 
× 100% 9 × 25 Gain % = 100 = 11 1 % 9 9
- The cost price of two dozen bananas is $ 32. After selling 18 bananas at the rate of $ 12 per dozen, the shopkeeper reduced the rate to $ 4 per dozen. The percent loss is
-
View Hint View Answer Discuss in Forum
Given that ,
Total C.P. = $ 32
Total S.P. = $ (18 + 2) = $ 20
Loss = $ (32 - 20) = $ 12∴ Loss percent = Loss × 100 Total C.P.
Correct Option: D
Given that ,
Total C.P. = $ 32
Total S.P. = $ (18 + 2) = $ 20
Loss = $ (32 - 20) = $ 12∴ Loss percent = Loss × 100 Total C.P. Loss percent = 12 × 100 = 37.5% 32
- Some articles were bought at 6 for $ 5, and sold at 5 for $ 6. Gain percent is :
-
View Hint View Answer Discuss in Forum
Let number of articles bought = 6 × 5 = 30
C.P. of 30 articles = $ 
5 × 30 
= $ 25 6 S.P. of 30 articles = $ 
6 × 30 
= $ 36 5
Gain = S.P. - C.P. = 36 - 25 = $ 11∴ Gain % = 11 × 100 = 44% 25
We can find required answer with the help of given formula :
Here, a = 6, p = 5 and b = 5, q = 6Gain % = 
aq - bp 
× 100% bp
Correct Option: D
Let number of articles bought = 6 × 5 = 30
C.P. of 30 articles = $ 
5 × 30 
= $ 25 6 S.P. of 30 articles = $ 
6 × 30 
= $ 36 5
Gain = S.P. - C.P. = 36 - 25 = $ 11∴ Gain % = 11 × 100 = 44% 25
We can find required answer with the help of given formula :
Here, a = 6, p = 5 and b = 5, q = 6Gain % = 
aq - bp 
× 100% bp Gain % = 
6 × 6 - 5 × 5 
× 100% 5 × 5 Gain % = 
36 - 25 
× 100% 25 Gain % = 11 × 100% = 44% 25

