Compound Interest
- A money-lender borrows money at 4% per annum and pays the interest at the end of the year. He lends it at 6% per annum compound interest compounded half yearly and receives the interest at the end of the year. In this way, he gains ₹ 104.50 a year. The amount of money he borrows, is
-
View Hint View Answer Discuss in Forum
Let the borrowed amount be y
According to the question,y 

1 + 3 
2 − 1 
− y × 4 × 1 = 104.50 100 100
[∵ Interest is compounded half yearly]
⇒ y [(1.03)2 – 1] – 0.04y = 104.50
Correct Option: C
Let the borrowed amount be y
According to the question,y 

1 + 3 
2 − 1 
− y × 4 × 1 = 104.50 100 100
[∵ Interest is compounded half yearly]
⇒ y [(1.03)2 – 1] – 0.04y = 104.50
⇒ 0.0609y – 0.04y = 104.50
⇒ 0.0209y = 104.5⇒ y = 104.5 = ₹ 5000 0.0209
- A person deposited a sum of ₹ 6,000 in a bank at 5% per annum simple interest. Another
person deposited ₹ 5,000 at 8% per annum compound interest. After two years, the difference of their interests will be
-
View Hint View Answer Discuss in Forum
Here , sum = ₹ 6,000 , Rate = 5% per annum , T = 2 years
S.I.= 6000 × 5 × 2 = ₹ 600 100 C.I. = 5000 

1 + 8 
2 − 1 
100 C.I. = 5000 

27 
2 − 1 
25
Correct Option: B
Here , sum = ₹ 6,000 , Rate = 5% per annum , T = 2 years
S.I.= 6000 × 5 × 2 = ₹ 600 100 C.I. = 5000 

1 + 8 
2 − 1 
100 C.I. = 5000 

27 
2 − 1 
25 C.I. = 5000 

729 − 625 

625 C.I. = 5000 × 104 = ₹ 832 625
∴ Required difference = C.I. - S.I.
∴ Required difference = ₹ (832–600) = ₹ 232
- The income of a company increases 20% per year. If the income is Rs. 26,64,000 in the year
2012, then its income in the year 2010 was :
-
View Hint View Answer Discuss in Forum
Given in question , Income = Rs. 26,64,000 , Rate = 20%
Let the income of company in 2010 be Rs. P
According to the question,A = P 
1 + R 
T 100 ⇒ 2664000 = P 
1 + 20 
2 100 ⇒ 2664000 = P 
1 + 1 
2 5
Correct Option: B
Given in question , Income = Rs. 26,64,000 , Rate = 20%
Let the income of company in 2010 be Rs. P
According to the question,A = P 
1 + R 
T 100 ⇒ 2664000 = P 
1 + 20 
2 100 ⇒ 2664000 = P 
1 + 1 
2 5 ⇒ 2664000 = P × 
6 
2 5 ⇒ P = 2664000 × 5 × 5 = Rs. 1850000 6 × 6
- Mr. Dutta desired to deposit his retirement benefit of Rs. 3 lacs partly to a post office and partly to a bank at 10% and 6% interests respectively. If his monthly interest income was Rs. 2000, then the difference of his deposits in the post office and in the bank was :
-
View Hint View Answer Discuss in Forum
Let the amount deposited in Post Office be Rs. y lakhs.
∴ Amount deposited in bank = Rs. (3 – y) lakhs
According to the question,y × 10 × 1 + (3 – y) × 6 × 1 100 × 12 100 × 12 = 2000 = 1 100000 50 ⇒ 10y + 18 – 6y = 1 × 1200 = 24 50
⇒ 4y = 24 – 18 = 6
Correct Option: C
Let the amount deposited in Post Office be Rs. y lakhs.
∴ Amount deposited in bank = Rs. (3 – y) lakhs
According to the question,y × 10 × 1 + (3 – y) × 6 × 1 100 × 12 100 × 12 = 2000 = 1 100000 50 ⇒ 10y + 18 – 6y = 1 × 1200 = 24 50
⇒ 4y = 24 – 18 = 6⇒ y = 6 = Rs. 3 lakhs 4 2
∴ Required difference = 0
- A sum of money is paid back in two annual instalments of Rs. 17, 640 each, allowing 5% compound interest compounded annually. The sum borrowed was
-
View Hint View Answer Discuss in Forum
According to question ,
Sum borrowed = Present worth of Rs. 17640 due 1 year hence + Present worth of Rs. 17640 due 2 years hence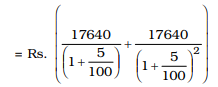
= Rs. 
17640 × 20 + 17640 × 20 × 20 
21 21 21
Correct Option: A
According to question ,
Sum borrowed = Present worth of Rs. 17640 due 1 year hence + Present worth of Rs. 17640 due 2 years hence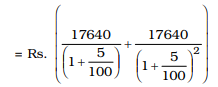
= Rs. 
17640 × 20 + 17640 × 20 × 20 
21 21 21
sum borrowed = Rs. (16800 + 16000) = Rs. 32800

