161. In order to have maximum power from a Pelton turbine, the bucket speed must be

1. 1. equal to the jet speed

   
   
   

   2. equal to half of the jet speed

   
   
   

   3. equal to twice the jet speed

   
   
   

   4. independent of the jet speed

   
   
   

---

1. View Hint View Answer Discuss in Forum

   For a pelton wheel, maximum power is obtained at,
   

   u =	υ
   	2

   Correct Option: B

   For a pelton wheel, maximum power is obtained at,
   

   u =	υ
   	2

---

162. The velocity triangles at the inlet and exit of the rotor of a turbo machine are shown. V denotes the absolute velocity of the fluid, W denotes the relative velocity of the fluid and U denotes the blade velocity. Subscripts 1 and 2 refer to inlet and outlet respectively If V₂ = W₁ and V₁ = W₂, then the degree of reaction is
     

1. 1. 0

   
   
   

   2. 1

   
   
   

   3. 0.5

   
   
   

   4. 0.25

   
   
   

---

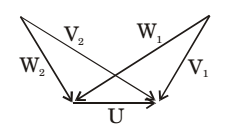
1. View Hint View Answer Discuss in Forum

   DOR =	W2² −W1²
   	2/W2² −W1²

   
   

   =	1	= 0.5
   	2

   Correct Option: C

   DOR =	W2² −W1²
   	2/W2² −W1²

   
   

   =	1	= 0.5
   	2

---

---

163. A hydraulic turbine develops 1000 kW power for a head of 40 m. If the head is reduced to 20 m, the power developed (in kW) is

1. 1. 177

   
   
   

   2. 354

   
   
   

   3. 500

   
   
   

   4. 707

   
   
   

---

1. View Hint View Answer Discuss in Forum

   Pu =	P
   	H3/2

   
   

   ∴	P1	=	P2
   	H13/2		H23/2

   
   ⇒  P₂ = 353.55 kW.

   Correct Option: B

   Pu =	P
   	H3/2

   
   

   ∴	P1	=	P2
   	H13/2		H23/2

   
   ⇒  P₂ = 353.55 kW.

---

164. A model of a hydraulic turbine is tested at a head of 1/4th of that under which the full scale turbine works. The diameter of the model is half of that of the full scale turbine. If N is the RPM of the full scale turbine, then the RPM of the model will be

1. 1. N/4

   
   
   

   2. N/2

   
   
   

   3. N

   
   
   

   4. 2N

   
   
   

---

1. View Hint View Answer Discuss in Forum

   Given data:
   

   Model: Hm =	Hp	;Dm =	Dp
   	4		9

   
   N_m = ?
   Prototype (Full scale turbine)
   N_p = N
   

   CH =	H
   	(ND)2

   
   (C_H)_model = (C_H)_prototype
   

   Hm	=	Hp
   Nm²Dm²		Np²Dp²

   
   

   Hp/4	=	Hp
   Nm² ×(Dp²/4)		Np²Dp²

   
   N_m = N

   Correct Option: C

   Given data:
   

   Model: Hm =	Hp	;Dm =	Dp
   	4		9

   
   N_m = ?
   Prototype (Full scale turbine)
   N_p = N
   

   CH =	H
   	(ND)2

   
   (C_H)_model = (C_H)_prototype
   

   Hm	=	Hp
   Nm²Dm²		Np²Dp²

   
   

   Hp/4	=	Hp
   Nm² ×(Dp²/4)		Np²Dp²

   
   N_m = N

---

---

165. The inlet angle of runner blades of a Francis turbine is 90°. The blades are so shaped that the tangential component of velocity at blade outlet is zero. The flow velocity remains constant through out the blade passage and is equal to half of the blade velocity at runner inlet. The blade efficiency of the runner is

1. 1. 25%

   
   
   

   2. 50%

   
   
   

   3. 80%

   
   
   

   4. 89%

   
   
   

---

1. View Hint View Answer Discuss in Forum

   Given data:
   B_i = 90°
   V_wo = 0
   

   Vfi = Vfo =	Ui
   	2

   
   
   Blade efficiency of the runner,
   

   ηH =	Vwiui	=	U1²	∴  Vwi = ui
   	gH		gH

   
   By energy balance equation,
   

   ρQgH = ρQVwiUi +	ρQV0²
   	2

   
   

   gH = Ui² +	Vfo²	Ui² +	Ui²
   	2		8

   
   

   gH =	9	Ui²
   	8

   
   

   ∴  ηH =	Ui²	=	8	= 0.8888
   	(9/8)Ui²		9

   
   = 88.88% ≈ 98%

   Correct Option: D

   Given data:
   B_i = 90°
   V_wo = 0
   

   Vfi = Vfo =	Ui
   	2

   
   
   Blade efficiency of the runner,
   

   ηH =	Vwiui	=	U1²	∴  Vwi = ui
   	gH		gH

   
   By energy balance equation,
   

   ρQgH = ρQVwiUi +	ρQV0²
   	2

   
   

   gH = Ui² +	Vfo²	Ui² +	Ui²
   	2		8

   
   

   gH =	9	Ui²
   	8

   
   

   ∴  ηH =	Ui²	=	8	= 0.8888
   	(9/8)Ui²		9

   
   = 88.88% ≈ 98%

---