116. A phenomenon is modeled using-n dimensional variables with k primary dimensions. The number of non-dimensional variables is

1. 1. k

   
   
   

   2. n

   
   
   

   3. n - k

   
   
   

   4. n + k

   
   
   

---

1. View Hint View Answer Discuss in Forum

   NA

   Correct Option: C

   NA

---

117. The parameters which determines the friction factor for turbulent flow in a rough pipe are

1. 1. Froude number and relative roughness

   
   
   

   2. Froude number and Mach number

   
   
   

   3. Reynolds number and relative roughness

   
   
   

   4. Mach number and relative roughness

   
   
   

---

1. View Hint View Answer Discuss in Forum

   NA

   Correct Option: C

   NA

---

---

118. A test is conducted on a one-fifth scale model of a Francis turbine under a head of 2 m and volumetric flow rate of 1 m³/s at 450 rpm. Take the water density and the acceleration due to gravity as 10³ kg/m³ and 10 m/s², respectively. Assume no losses both in model and prototype turbines. The power (in MW) of a full sized turbine while working under a head of 30 m is _______ (correct to two decimal places).

1. 1. 29.25

   
   
   

   2. 30.26

   
   
   

   3. 29.05

   
   
   

   4. 29

   
   
   

---

1. View Hint View Answer Discuss in Forum

   	Dm	=	1
   	Dp		5

   
   Model: H = 2 m, Q = 1 m³ /s
   Prototype: H = 30 m, P =?
   N = 450 rpm, ρ = 1000 kg/m³ /g = 10 m/s²
   For model, pm = ρgQH = 1000 × 10 × 1 × 2 = 20 kW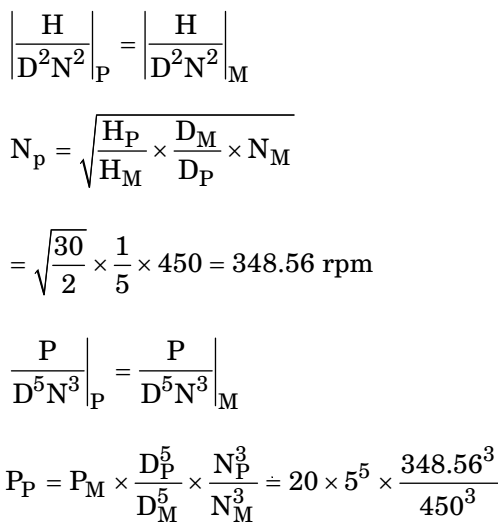
   
   = 29045.24 kW
   = 29.05 mW

   Correct Option: C

   	Dm	=	1
   	Dp		5

   
   Model: H = 2 m, Q = 1 m³ /s
   Prototype: H = 30 m, P =?
   N = 450 rpm, ρ = 1000 kg/m³ /g = 10 m/s²
   For model, pm = ρgQH = 1000 × 10 × 1 × 2 = 20 kW
   
   = 29045.24 kW
   = 29.05 mW

---

119. For a Pelton wheel with a given water jet velocity, the maximum output power from the Pelton wheel is obtained when the ratio of the bucket speed to the water jet speed is ______ (correct to two decimal places).

1. 1. u =	v
      	2

   
   
   

   2. u =	2
      	v

   
   
   

   3. v =	u
      	2

   
   
   

   4. None of these

   
   
   

---

1. View Hint View Answer Discuss in Forum

   In pelton wheel:-
   

   η=	2(vu -u2)
   	v2

   
   where,
   u = bucket speed
   v = water jet speed
   So, For maximum efficiency:-
   

   	dx	= 0
   	du

   
   2(v – 2u) = 0 v = 2u
   

   u =	v
   	2

   Correct Option: A

   In pelton wheel:-
   

   η=	2(vu -u2)
   	v2

   
   where,
   u = bucket speed
   v = water jet speed
   So, For maximum efficiency:-
   

   	dx	= 0
   	du

   
   2(v – 2u) = 0 v = 2u
   

   u =	v
   	2

---

---

120. Which one of the following statements is TRUE?

1. 1. Both Pelton and Francis turbines are impulse turbines.

   
   
   

   2. Francis turbine is a reaction turbine but Kaplan turbine is an impulse turbine.

   
   
   

   3. Francis turbine is an axial-flow reaction turbine.

   
   
   

   4. Kaplan turbine is an axial-flow reaction turbine.

   
   
   

---

1. View Hint View Answer Discuss in Forum

   Kaplan turbine is an axial-flow reaction turbine

   Correct Option: D

   Kaplan turbine is an axial-flow reaction turbine

---