Simplification
- The simplified value of
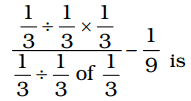
-
View Hint View Answer Discuss in Forum
The given expression

Correct Option: A
The given expression

- The value of 1 ÷ [1 + 1 ÷ {1 + 1 ÷ (1 + 1 ÷ 2)}] is
-
View Hint View Answer Discuss in Forum
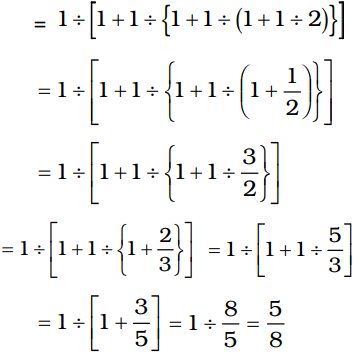
Correct Option: B
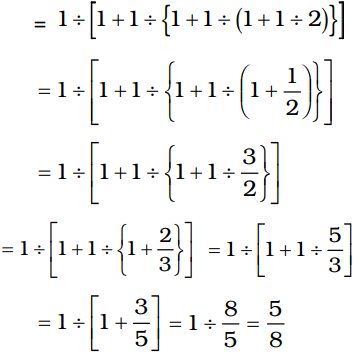
-
If I = 3 ÷ 5 , II = 3 ÷ [(4 ÷ 5) ÷ 6], 4 6
III = [3 ÷ (4 ÷ 5)] ÷ 6, IV = 3 ÷ 4 (5 ÷ 6) then
-
View Hint View Answer Discuss in Forum
I. = 3 × 6 = 9 4 5 10 II. = 3 ÷ 
4 × 1 
= 3 ÷ 2 = 45 5 6 15 2 III. = 
3 ÷ 4 
÷ 6 = 15 ÷ 6 = 5 5 4 8 IV. = 3 ÷ 4 × 5 = 3 ÷ 10 = 9 6 3 10
Obviously, (I) and (IV) are equalCorrect Option: B
I. = 3 × 6 = 9 4 5 10 II. = 3 ÷ 
4 × 1 
= 3 ÷ 2 = 45 5 6 15 2 III. = 
3 ÷ 4 
÷ 6 = 15 ÷ 6 = 5 5 4 8 IV. = 3 ÷ 4 × 5 = 3 ÷ 10 = 9 6 3 10
Obviously, (I) and (IV) are equal
-
1 + 1 + 1 + 1 + 1 + 1 = ? 30 42 56 72 90 110
-
View Hint View Answer Discuss in Forum
1 + 1 + 1 + 1 + 1 + 1 5 × 6 6 × 7 7 × 8 8 × 9 9 × 10 10 × 11 = 1 − 1 + 1 − 1 + 1 − 1 + 1 − 1 + 1 − 1 + 1 − 1 5 6 6 7 7 8 8 9 9 10 10 11 = 1 − 1 = 11 − 5 = 6 5 11 55 55 Correct Option: D
1 + 1 + 1 + 1 + 1 + 1 5 × 6 6 × 7 7 × 8 8 × 9 9 × 10 10 × 11 = 1 − 1 + 1 − 1 + 1 − 1 + 1 − 1 + 1 − 1 + 1 − 1 5 6 6 7 7 8 8 9 9 10 10 11 = 1 − 1 = 11 − 5 = 6 5 11 55 55
- The value of
is0.1 × 0.1 × 0.1 + 0.2 ×0.2 × 0.2 + 0.3 × 0.3 × 0.3 − 3 × 0.1 × 0.2 × 0.3 0.1 × 0.1 + 0.2 × 0.2 + 0.3 × 0.3 − 0.1 × 0.2 − 0.2 × 0.3 − 0.3 × 0.1
-
View Hint View Answer Discuss in Forum
Using (x) of Basic Formulae
Let 0.1 = a, 0.2 = b and 0.3 = c
Then, we have,a × a × a + b × b × b + c × c × c − 3abc a × a + b × b + c × c − ab − bc − ac = a3 + b3 + c3 − 3abc a2 + b2 + c2 − ab − bc − ac
= a + b + c
= 0.1 + 0.2 + 0.3 = 0.6Correct Option: B
Using (x) of Basic Formulae
Let 0.1 = a, 0.2 = b and 0.3 = c
Then, we have,a × a × a + b × b × b + c × c × c − 3abc a × a + b × b + c × c − ab − bc − ac = a3 + b3 + c3 − 3abc a2 + b2 + c2 − ab − bc − ac
= a + b + c
= 0.1 + 0.2 + 0.3 = 0.6

