LCM and HCF
- Find the greatest number which will exactly divide 200 and 320.
-
View Hint View Answer Discuss in Forum
Firstly , We find HCF of 200 and 320

Required number = HCF of 200 and 320Correct Option: D
Required number = HCF of 200 and 320
Firstly , We find HCF of 200 and 320
Required number = HCF of 200 and 320 = 40
- The greatest number that divides 411, 684, 821 and leaves 3, 4 and 5 as remainders, respectively, is
-
View Hint View Answer Discuss in Forum
We know that the largest number which when divide the numbers a, b and c give remainders as p, q, r respectively is given by H.C.F. of (a – p), (b – q) and (c – r).
Required number = HCF of 411 – 3 = 408; 684 – 4 = 680 and 821 – 5 = 816
HCF of 408 and 816 = 408
HCF of 408 and 680
∴ Required number = HCF of 408 and 680Correct Option: C
We know that the largest number which when divide the numbers a, b and c give remainders as p, q, r respectively is given by H.C.F. of (a – p), (b – q) and (c – r).
Required number = HCF of 411 – 3 = 408; 684 – 4 = 680 and 821 – 5 = 816
HCF of 408 and 816 = 408
HCF of 408 and 680
∴ Required number = HCF of 408 and 680
Hence , Required number = 136
- Which greatest number will divide 3026 and 5053 leaving remainders 11 and 13 respectively?
-
View Hint View Answer Discuss in Forum
We know that the largest number which when divide the numbers a, b and c give remainders as p, q, r respectively is given by H.C.F. of (a – p), (b – q) and (c – r).
3026 –11 = 3015 and 5053 –13 = 5040
Required number = HCF of 3015 and 5040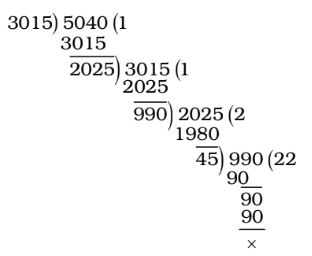
Correct Option: C
We know that the largest number which when divide the numbers a, b and c give remainders as p, q, r respectively is given by H.C.F. of (a – p), (b – q) and (c – r).
3026 –11 = 3015 and 5053 –13 = 5040
Required number = HCF of 3015 and 5040
∴ Required number = HCF of 3015 and 5040 = 45
- What is the greatest number that will divide 307 and 330 leaving remainders 3 and 7 respectively ?
-
View Hint View Answer Discuss in Forum
As we know that the largest number which when divide the numbers a, b and c give remainders as p, q, r respectively is given by H.C.F. of (a – p), (b – q) and (c – r).
The number will be HCF of 307 – 3 = 304 and 330 – 7 = 323.
Correct Option: A
As we know that the largest number which when divide the numbers a, b and c give remainders as p, q, r respectively is given by H.C.F. of (a – p), (b – q) and (c – r).
The number will be HCF of 307 – 3 = 304 and 330 – 7 = 323.
∴ Required number = 19
- Let N be the greatest number that will divide 1305, 4665 and 6905 leaving the same remainder in each case. Then, sum of the digits in N is :
-
View Hint View Answer Discuss in Forum
We can say that the largest number which when divide the numbers a, b and c give remainders as p, q, r respectively is given by H.C.F. of (a – p), (b – q) and (c – r).
The greatest number N = HCF of (1305 – t ), (4665 – t ) and (6905 – t), where t is the remainder
= HCF of (4665 – 1305), (6905– 4665) and (6905 – 1305)
= HCF of 3360, 2240 and 5600
Correct Option: A
We can say that the largest number which when divide the numbers a, b and c give remainders as p, q, r respectively is given by H.C.F. of (a – p), (b – q) and (c – r).
The greatest number N = HCF of (1305 – t ), (4665 – t ) and (6905 – t), where t is the remainder
= HCF of (4665 – 1305), (6905– 4665) and (6905 – 1305)
= HCF of 3360, 2240 and 5600
∴ N = 1120
Sum of digits = 1 + 1 + 2 + 0 = 4

