-
ABC is an isosceles triangle with AB = AC. The side BA is produced to D such that AB = AD. If ∠ABC = 30°, then ∠BCD is equal to
-
- 45°
- 90°
- 30°
- 60°
- 45°
Correct Option: B
We draw a figure of an isosceles triangle ABC whose side BA is produced to D such that AB = AD , 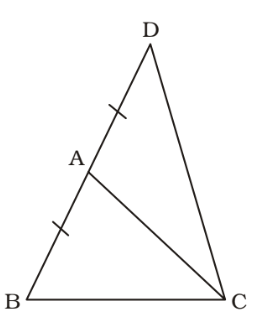
Given , AB = AC = AD
⇒ ∠ABC = ∠ACB = 30°
⇒ ∠BAC = 180° – 60° = 120°
Now, ∠DAC = 180° – 120° = 60°
⇒ ∠ADC + ∠ACD = 120°
∴ ∠ACD = 120 7divide; 2 = 60°
∴ ∠BCD = ∠ACB + ∠ACD
∴ ∠BCD = 30° + 60° = 90°

