-
ABC is a triangle. The bisectors of the internal angle ∠B and external angle ∠C intersect at D. If ∠BDC= 50°, then ∠A is
-
- 100°
- 90°
- 120°
- 60°
- 100°
Correct Option: A
As per the given in question , we draw a figure of triangle ABC in which bisectors of the internal angle ∠B and external angle ∠C intersect at point D , 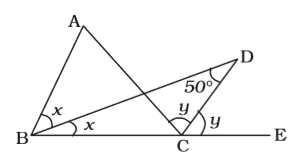
[Exterior angle is sum of opp. interior angles]
∠ACE = ∠BAC + ∠ABC
⇒ 2y = ∠A + 2x
Similarly ,
∠DCE = ∠DBC + ∠BDC
⇒ y = 50° + x
⇒ ∠A = 2y – 2x = 100 + 2x – 2x = 100°

