-
Two chords of length a unit and b unit of a circle make angles 60° and 90° at the centre of a circle respectively, then the correct relation is
-
-
b = 3 a 2 - b = √2a
- b = 2a
- b = √3a
-
Correct Option: B
As per the given in question , we draw a figure of circle with centre O in which two chords of length a unit and b unit , 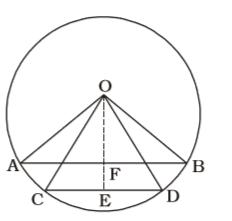
radius(r) = OA = OB = OC = OD
∠OAB = 90°; AB = b, CD = a
From ∆ OAB,
OA² + OB² = AB²
⇒ r² + r² = b²
⇒ 2r² = b²
| ⇒ r² = | |
| 2 |
| ⇒ r = | .......(ii) | |
| √2 |
In ∆ OCD,
∠COD = 60° ;
∴ ∠OCD = ∠ODC = 60°
∴ OC = CD
⇒ r = a ......(ii)
From equations (i) and (ii),
| = a ⇒ b = √2a | |
| √2 |

