Alligation or Mixture
- In what ratio must water be added to spirit to gain 25% by selling it at cost price?
-
View Hint View Answer Discuss in Forum
Let cost price of spirit be Re. 1 per liter.
Then SP of mixture = Re. 1 per liter
Gain = 25%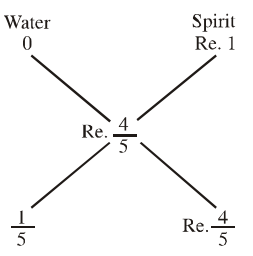
Correct Option: A
Let cost price of spirit be Re. 1 per liter.
Then SP of mixture = Re. 1 per liter
Gain = 25%
So, CP of mixture = 1 × (100 / 125) = Re. 4 / 5
We assume that CP of water is zero.
Using allegation rule on cost price,
Water should be mixed to spirit in the ratio (1 / 5) : (4 / 5) or 1 : 4
- 6 liters of milk and water mixture has 75% milk in it. How much milk should be added to the mixture to make it 90% pure?
-
View Hint View Answer Discuss in Forum
The given solution has 75% milk.
Milk to be added has 100% milk.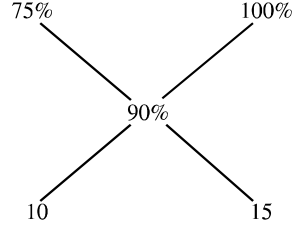
Correct Option: B
The given solution has 75% milk.
Milk to be added has 100% milk.
Milk should be added to the given mixture in the ratio 15 : 10 or 3 : 2
∴ Quantity of milk to be added = (3 / 2) × 6 = 9 liters.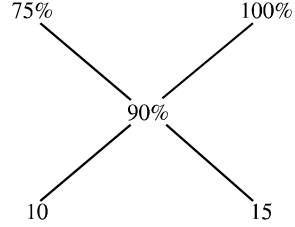
- Two vessels A and B contain mixture of milk and water in the ratio 4 : 1 and 9 : 11 respectively. They are mixed in the ratio of 3 : 2. Find the ratio of milk and water in the resulting mixture.
-
View Hint View Answer Discuss in Forum
Fraction is Milk Water A: 4 / 5 1 / 5 B: 9 / 20 11 / 20 (3A + 2B) = A and B: ((12 / 5) + (9 / 10)) ((3 / 5) + (11 / 10))
Correct Option: B
Fraction is Milk Water A: 4 / 5 1 / 5 B: 9 / 20 11 / 20 (3A + 2B) = A and B: ((12 / 5) + (9 / 10)) ((3 / 5) + (11 / 10))
(33 / 10) (17 / 10)
So, Ratio of milk: water in the resulting mixture = 33: 17.
- A shopkeeper buys 26 liter of milk @ Rs. 16 per liter. He also buys from another source an inferior quality of milk @ Rs. 10 per liter. How much quantity of the later should he buy to mix it with the former so that he can sell the mixture @ Rs. 14 per liter without making any loss?
-
View Hint View Answer Discuss in Forum
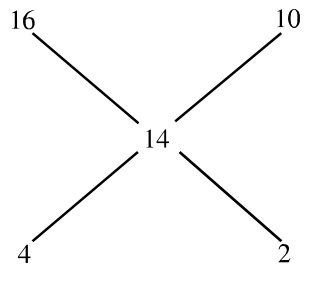
Quantity of milk @ Rs.10 per liter/ Quantity of milk @ Rs. 16 per liter= 1 / 2
Correct Option: A
Quantity of milk @ Rs.10 per liter / Quantity of milk @ Rs. 16 per liter = 1 / 2
So, quantity of milk @ Rs. 10 per liter = 26 / 2 = 13 liter.
2nd Method
Let us assume shopkeeper buy P liter milk of price @ Rs. 10 per liter.
Buy price of 26 liter of milk @ Rs. 16 per liter = 26 x 16
Buy price of P liter of milk @ Rs. 10 per liter = P x 10
Sell price of total milk ( P + 26 ) @ Rs. 14 per liter = 14 x ( P + 26 )
According to question there is no loss or no profit.
Then Buy Price = Sell Price
26 x 16 + P x 10 = 14 x ( P + 26 )
⇒ 26 x 16 + 10P = 14P + 14 x 26
⇒ 26 x 16 - 26 x 14 = 14P - 10P
⇒ 2 x 26 = 4P
⇒ 4P = 2 x 26
⇒ P = 2 x 26 / 4 = 13
So, quantity of milk @ Rs. 10 per liter = 13 liter.
- 640 ml of a mixture contains milk and water in ratio 6:2. How much of the water is to be added to get a new mixture containing half milk and half water ?
-
View Hint View Answer Discuss in Forum
Let us assume the ratio factor is Q.
According to question,
Quantity of Milk in mixture = 6Q ml
Quantity of Water in mixture = 2Q ml
Quantity of Milk in mixture + Quantity of Water in mixture = 640
Proceed and solve the equation as per given question.Correct Option: B
Let us assume the ratio product is Q.
According to question,
Quantity of Milk in mixture = 6Q ml
Quantity of Water in mixture = 2Q ml
Quantity of Milk in mixture + Quantity of Water in mixture = 640
6Q + 2Q = 640
8Q = 640
Q = 640/8
Q = 80
Quantity of Milk in mixture = 6Q ml = 6 x 80 = 480 ml
Quantity of Water in mixture = 2Q ml = 2 x 80 = 160 ml
To find the new mixture containing half milk and half water,
Quantity of water to be added for making the mixture of equal quantity of milk and water = 480 - 160 = 320

