Engineering Mathematics Miscellaneous Easy Questions and Answers | Page - 10

Engineering Mathematics Miscellaneous

A calculator has accuracy up to 8 digits after decimal place. The value of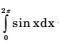

when evaluated using this calculator by trapezoidal method with 8 equal intervals, to 5 significant digits is

1. 0.00000
1. 1.0000
1. 0.00500
1. 0.00025

View Hint View Answer Discuss in Forum

h = 2π - 0 = π
8 4

y₀ = sin (0) = 0
y₁ = sin π = 0.7071
4

y₂ = sin π = 1
2

y₃ = sin 3π = 0.7071
4

y₄ = sin (π) = 0
y₅ = sin 5π = -0.7071
4

y₆ = sin 6π = -1
4

y₇ = sin 7π = -0.7071
4

y₈ = sin 8π = 0
4

Trapezoidal rule
h [ y₀ + y_n + 2(y₁ + y₂ + ... + y_{n - 1}) ]
2

[(0 + 0) + 2 (0.7071 + 1 + .7071 + -0.7071 - 0.7071)] = 0

Correct Option: A

h = 2π - 0 = π
8 4

y₀ = sin (0) = 0
y₁ = sin π = 0.7071
4

y₂ = sin π = 1
2

y₃ = sin 3π = 0.7071
4

y₄ = sin (π) = 0
y₅ = sin 5π = -0.7071
4

y₆ = sin 6π = -1
4

y₇ = sin 7π = -0.7071
4

y₈ = sin 8π = 0
4

Trapezoidal rule
h [ y₀ + y_n + 2(y₁ + y₂ + ... + y_{n - 1}) ]
2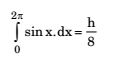

[(0 + 0) + 2 (0.7071 + 1 + .7071 + -0.7071 - 0.7071)] = 0

An analytic function of a complex variable z = x + iy is expressed as f(z) = u(x, y)+ iv(x, y), where i = √-1. If u(x, y) = x² - y², then expression for v(x, y) in terms of x, y and a general constant c would be

1. xy + c
1. x² + y² + c
  2
1. 2xy + c
1. (x - y)² + c
  2

View Hint View Answer Discuss in Forum

Given f(z) = μx(x, y) + iv (x, y) is analytic and x = x² - y²
We know that if f(z) = μ + iv is analytic then C-R equations will be satisfied.
i.e. ∂μ = ∂v and ∂μ = - ∂v
∂x ∂y ∂y ∂x

∴ v = 2xy + c is correct answer

Correct Option: C

Given f(z) = μx(x, y) + iv (x, y) is analytic and x = x² - y²
We know that if f(z) = μ + iv is analytic then C-R equations will be satisfied.
i.e. ∂μ = ∂v and ∂μ = - ∂v
∂x ∂y ∂y ∂x

∴ v = 2xy + c is correct answer

An analytic function of a complex variable z = x + iy is expressed as f(z) = u(x, y)+ iv(x, y), where i = √-1. If u(x, y) = 2xy, then v(x, y) must be

1. x² + y² + constant
1. x² - y² + constant
1. -x² + y² + constant
1. -x² - y² + constant

View Hint View Answer Discuss in Forum

Given f(z) = u + iv is analytic and u = 2xy
We know that if f(z) is analytic then CR equations will be satisfied.
i.e. ∂u = ∂v and ∂u = - ∂v
∂x ∂y ∂y ∂x

Verify the options which satisfy the above C-R equations.
v = – x² + y² + constant, satisfies the C-R equation.

Correct Option: C

Given f(z) = u + iv is analytic and u = 2xy
We know that if f(z) is analytic then CR equations will be satisfied.
i.e. ∂u = ∂v and ∂u = - ∂v
∂x ∂y ∂y ∂x

Verify the options which satisfy the above C-R equations.
v = – x² + y² + constant, satisfies the C-R equation.

If(z) = u(x, y) + iv(x, y) is an analytic function or complex variable z = x + iy where i = √-1 , u (x, y) = 2 xy, then v(x, y) may be expressed as

1. -x² + y² + constant
1. x² - y² + constant
1. x² + y² + constant
1. -(x² + y²) + constant

View Hint View Answer Discuss in Forum

Given f(z) = u + iv is analytic and u = 2xy We know that if f(z) is analytic then CR equations will be satisfied.
i.e. ∂u = ∂v
∂x ∂y

and ∂u = - ∂v
∂y ∂x

Verify the options which satisfy the above C-R equations.
v = – x² + y² + constant, satisfies the C-R equation.

Correct Option: A

Given f(z) = u + iv is analytic and u = 2xy We know that if f(z) is analytic then CR equations will be satisfied.
i.e. ∂u = ∂v
∂x ∂y

and ∂u = - ∂v
∂y ∂x

Verify the options which satisfy the above C-R equations.
v = – x² + y² + constant, satisfies the C-R equation.

An analytic function of a complex variable z = x + iy is expressed as f(z) = u(x, y) + i v(x, y) where i = √-1. If u = xy, the expression for v should be

1. (x + y)² + k
  2
1. x² - y² + k
  2
1. y² - x² + k
  2
1. (x - y)² + k
  2

View Hint View Answer Discuss in Forum

Given u = x,y
For analytic function
∂u = ∂v
∂x ∂y

and ∂u = - ∂v
∂y ∂x

By Milne Thomson method
Let w = u + zv
∴ dw = ∂y + i ∂v
dz ∂x ∂x

= ∂u - i ∂u
∂x ∂y

or dw = y - ix
dz

Replacing x by z and y by 0, we get
dw = 0 - iz
dz

where , z = x + iy
∴ dw = - iz dz
Integrating , w = -i z² + C
2

where C is a constant ,
∴ V = Im -i z² + C
2

= Im -i (x² - y² + 2ixy) + C
2

or V = y² - x²
2

Correct Option: C

Given u = x,y
For analytic function
∂u = ∂v
∂x ∂y

and ∂u = - ∂v
∂y ∂x

By Milne Thomson method
Let w = u + zv
∴ dw = ∂y + i ∂v
dz ∂x ∂x

= ∂u - i ∂u
∂x ∂y

or dw = y - ix
dz

Replacing x by z and y by 0, we get
dw = 0 - iz
dz

where , z = x + iy
∴ dw = - iz dz
Integrating , w = -i z² + C
2

where C is a constant ,
∴ V = Im -i z² + C
2

= Im -i (x² - y² + 2ixy) + C
2

or V = y² - x²
2