146. The value of ∮r	3z - 5	dz
     	(z - 1)(z - 2)

     
     along a closed path R is is equal to (4πi), where z = x + iy and i
     = √- 1 . The correct path r is

1. 1. 

   
   
   

   2. 

   
   
   

   3. 

   
   
   

   4. 

   
   
   

---

1. View Hint View Answer Discuss in Forum

   ∮r	3z - 5	dz = 4πi = 2πi (2)
   	(z - 1)(z - 2)

   
   Sum of residues must be equal to 2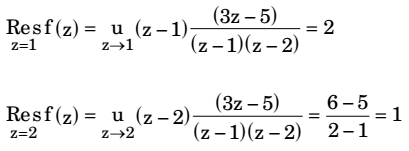
   
   There force z = 1 must lies inside C
   Z = 2 lies outside C

   Correct Option: B

   ∮r	3z - 5	dz = 4πi = 2πi (2)
   	(z - 1)(z - 2)

   
   Sum of residues must be equal to 2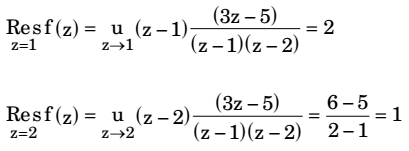
   
   There force z = 1 must lies inside C
   Z = 2 lies outside C

---

147. Consider a function u which depends on position x and time t. The partial differential equation
     

     	δu	=	δ2u	is known as the
     	δt		δx2

1. 1. Wave equation

   
   
   

   2. Heat equation

   
   
   

   3. Laplace's equation

   
   
   

   4. Elasticity equation

   
   
   

---

1. View Hint View Answer Discuss in Forum

   	δu	=	δ2u
   	δt		δx2

   
   is called as heat equation.

   Correct Option: B

   	δu	=	δ2u
   	δt		δx2

   
   is called as heat equation.

---

---

148. Consider the following partial differential equation u(x, y) with the constant c > 1:
     

     	δu	+ c	δu	= 0
     	δy		δx

     
     Solution of this equation is

1. 1. u(x, y) = f(x + cy)

   
   
   

   2. u(x, y) = f(x – cy)

   
   
   

   3. u(x, y) = f(cx + y)

   
   
   

   4. u(x, y) = f(cx – y)

   
   
   

---

1. View Hint View Answer Discuss in Forum

   u = f (x – cy)
   

   	δu	= f' (x - cy)(1)
   	δx

   
   

   	δu	= f' (x - cy)(-c)
   	δx

   
   = –c. f¹ (x–cy)
   

   = - c .	δu
   	δx

   
   

   ∴	δu	+ c	δu	= 0
   	δy		δx

   Correct Option: B

   u = f (x – cy)
   

   	δu	= f' (x - cy)(1)
   	δx

   
   

   	δu	= f' (x - cy)(-c)
   	δx

   
   = –c. f¹ (x–cy)
   

   = - c .	δu
   	δx

   
   

   ∴	δu	+ c	δu	= 0
   	δy		δx

---

149. The partial differential equation
     

     	δu	+ u	δu	=	δ2u	is
     	δt		δx		δx2

1. 1. linear equation of order 2

   
   
   

   2. non-linear equation of order 1

   
   
   

   3. linear equation of order 1

   
   
   

   4. non-linear equation of order 2

   
   
   

---

1. View Hint View Answer Discuss in Forum

   Order is index of a derivative present in a PDE (i.e.) maximum there.
   

   Hence,	δ2u	⇒ highest index 2 ⇒ order = 2
   	δn2

   
   if y, y₂ solution of a PDE, then PDE is linearly independent.
   

   	δy1	+	y1δy1	=	δy12	......(1)
   	δt		δx		δx2

   
   

   	δy2	+	y2δy2	=	δ2y2	......(2)
   	δt		δx		δx2

   
   If y = ay₁ + a₁ y₂ is a sum of the PDE
   

   	δ	(ay1 + a1y1) + (ay1 + a2y2)	δ	(ay1 + ay2) =	δ2	(ay1 + ay2)
   	δt		d2		d22

   
   Hence PDE is non linear of order 2

   Correct Option: D

   Order is index of a derivative present in a PDE (i.e.) maximum there.
   

   Hence,	δ2u	⇒ highest index 2 ⇒ order = 2
   	δn2

   
   if y, y₂ solution of a PDE, then PDE is linearly independent.
   

   	δy1	+	y1δy1	=	δy12	......(1)
   	δt		δx		δx2

   
   

   	δy2	+	y2δy2	=	δ2y2	......(2)
   	δt		δx		δx2

   
   If y = ay₁ + a₁ y₂ is a sum of the PDE
   

   	δ	(ay1 + a1y1) + (ay1 + a2y2)	δ	(ay1 + ay2) =	δ2	(ay1 + ay2)
   	δt		d2		d22

   
   Hence PDE is non linear of order 2

---

---

150. The Blasius equation,
     

     	d3f	+	f		d2f	= 0, is a
     	dη3		2		dη2

1. 1. second order nonlinear ordinary differential equation

   
   
   

   2. third order nonlinear ordinary differential equation

   
   
   

   3. third order linear ordinary differential equation

   
   
   

   4. mixed order nonlinear ordinary differential equation

   
   
   

---

1. View Hint View Answer Discuss in Forum

   According to Blasius equation,
   

   f" +	f	. f" = 0,
   	2

   
   is a third order linear ordinary differential equation.

   Correct Option: B

   According to Blasius equation,
   

   f" +	f	. f" = 0,
   	2

   
   is a third order linear ordinary differential equation.

---