Engineering Mathematics Miscellaneous
- Numerical integration using trapezoidal rule gives the best result for a single variable function, which is
-
View Hint View Answer Discuss in Forum
Trapezoidal rule gives best result when function is linear in nature.
Correct Option: A
Trapezoidal rule gives best result when function is linear in nature.
- The values of function f(x) at 5 discrete points are given below:
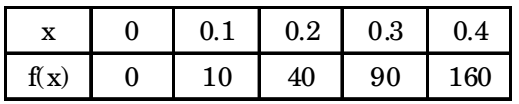
Using Trapezoidal rule step size of 0.1, the value of ∫0.40 f (x) dx is
-
View Hint View Answer Discuss in Forum
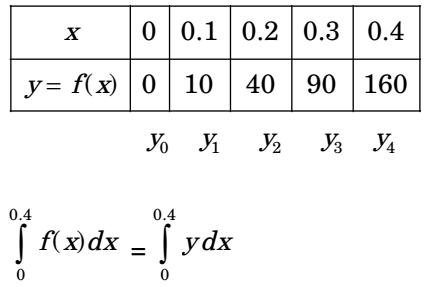
= h [ (y0 + y4) + 2(y1 + y2 + y3) ] 2 = 0.1 [ (0 + 160) + 2(10 + 40 + 90) ] = 22 2 Correct Option: D

= h [ (y0 + y4) + 2(y1 + y2 + y3) ] 2 = 0.1 [ (0 + 160) + 2(10 + 40 + 90) ] = 22 2
- Using a unit step size, the volume of integral ∫21 xlnxdx by trapezoidal rule is ______
-
View Hint View Answer Discuss in Forum

∴ I = h [ y0 + yn ] 2 I = 1 [ 0 + 2 In 2 ] 2
= In 2 = 0.693Correct Option: A

∴ I = h [ y0 + yn ] 2 I = 1 [ 0 + 2 In 2 ] 2
= In 2 = 0.693
- If x is the mean of data 3, x, 2 and 4, then the mode is
-
View Hint View Answer Discuss in Forum
3, x, 2 and 4
Mean, x = 3 + x + 2 + 4 4
⇒ 4x = 9 + x
⇒ 3x = 9 x = 3
So, 3, 3, 2 and 4
Mode = 3Correct Option: C
3, x, 2 and 4
Mean, x = 3 + x + 2 + 4 4
⇒ 4x = 9 + x
⇒ 3x = 9 x = 3
So, 3, 3, 2 and 4
Mode = 3
- Let X1 and X2 be two independent exponentially distributed random variables with means 0.5 and 0.25 respectively. Then Y = min (X1, X2) is
-
View Hint View Answer Discuss in Forum
Mean (X1) = 0.5
1 = 0.5 λ1 λ1 = 1 = 2 0.5
Mean (X2) = 0.251 = 0.25 ⇒ λ2 = 4 λ2
y= mean (X1, X2)Mean (y) = 1 = 1 = 1 y1 + y2 2 + 4 6 Correct Option: A
Mean (X1) = 0.5
1 = 0.5 λ1 λ1 = 1 = 2 0.5
Mean (X2) = 0.251 = 0.25 ⇒ λ2 = 4 λ2
y= mean (X1, X2)Mean (y) = 1 = 1 = 1 y1 + y2 2 + 4 6

