-
If the altitude of an equilateral triangle is 12√3 cm, then its area would be :
-
- 12 cm²
- 144√3 cm²
- 72 cm²
- 36√3 cm²
- 12 cm²
Correct Option: B
Using Rule 6, A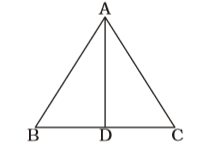
AD = 12√3 cm.
AB = 2x cm. (let)
BD = x cm.
From ∆ABD,
AD = √AB² - BD²
= √(2x)² - x²
= √4x² - x²
= √3x²
= √3x
∴ √3x = 12√3
⇒ x = 12 cm.
∴ AB = 2x = 2 × 12 = 24 cm.
| ∴ Area of ∆ABC = | × side² | |
| 4 |
| = | × 24 × 24 | |
| 4 |
= 144√3 sq. cm.

