Find the largest number of four digits such that on dividing

Home » Aptitude » LCM and HCF » Question

Find the largest number of four digits such that on dividing by 15, 18, 21 and 24 the remainders are 11, 14, 17 and 20 respectively.

1. 6557
2. 7556
3. 5675
4. 7664

Correct Option: B

As per the given details in question , we have
15 = 3 × 5
18 = 3² × 2
21 = 3 × 7
24 = 2³ × 3
LCM of 15 , 18 , 21 and 24 = 8 × 9 × 5 × 7 = 2520
The largest number of four digits = 9999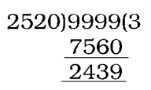

Required number = 9999 – 2439 – 4 = 7556
(Because 15 – 11 = 4
18 – 14 = 4
21 – 17 = 4
24 – 20 = 4)