-
The area of an isosceles trapezium is 176 cm² and the height is
2/11 th of the sum of its parallel sides. If the ratio of the length of the parallel sides is 4 : 7, then the length of a diagonal (in cm) is
-
- 28
- √137
- 2√137
- 24
- 28
Correct Option: C
Using Rule 13, 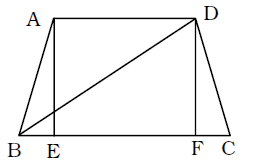
BC = 7x cm.
AD = 4x cm.
AB = DC ;
AE ⊥ BC ; DF ⊥ BC
Area of trapezium ABCD
| = | × (AD + BC) × AE | |
| 2 |
| ⇒ 176 = | × 11x × | × 11x | ||
| 2 | 11 |
⇒ 176 = 11x²
| ⇒ x² = | = 16 | |
| 11 |
⇒ x = √16 = 4
∴ BC = 7 × 4 = 28 cm.
AD = 4 × 4 = 16 cm.
| ∴ BE = FC = | (28 - 16) cm | |
| 2 |
= 6 cm.
∴ BF = 16 + 6 = 22 cm.
| ∴ DF = | × 11x = 2x | |
| 11 |
= 8 cm.
∴ Diagonal BD = √BF² + FB²
= √ 22² + 8²
= √484 + 64
= √548 = 4 √4 × 137
= 2√137 cm.

