-
The height of the cone is 30 cm. A small cone is cut off at the top by a plane parallel to its base. If its volume is 1/27 of the volume of the cone, at what height, above the base, is the section made?
-
- 6 cm
- 8 cm
- 10 cm
- 20 cm
- 6 cm
Correct Option: D
Let H and R be the height and radius of bigger cone respectively and h and r that of smaller cone.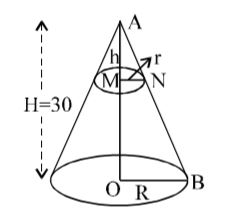
From triangles AOB and AMN. ∠A is common and MN || OB.
∴ Triangles AOB and AMN are similar,
| ∴ | = | ||
| AM | MN |
| ⇒ | = | .........(i) | ||
| h | r |
| ∴ Volume = | πr² × h | |
| 3 |
| Volume of bigger cone = | πR²H | |
| 3 |
According to the question,
| πr²h = |  | πR²H |  | × | |||
| 3 | 3 | 27 |
| ⇒ r²h = | ⇒ 27r²h = R²H | |
| 27 |
| ⇒ | = | ||
| H | r² |
| ⇒ | = |  |  | ² | From(i) | ||
| H | h |
| ⇒ | = | ||
| H | r² |
⇒ 27h³ = 900H = 900 × 30
| h³ = | = 1000 | |
| 27 |
⇒ h = 3√1000 = 10 cm
∴ Required height = 30 – 10 = 20 cm

