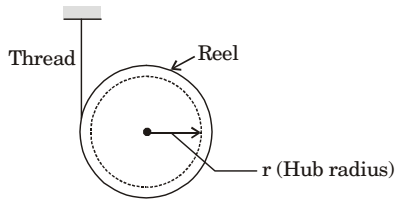
41. The tension in the thread is
    

1. 1. 	mgr²
      	(r² + k²)

   
   
   

   2. 	mgrk
      	(r² + k²)

   
   
   

   3. 	mgk²
      	(r² + k²)

   
   
   

   4. 	mg
      	(r² + k²)

      
      

   
   
   

---

1. View Hint View Answer Discuss in Forum

   Tension in thread,
   

   T =		mk²	×	gr²	=	mgk²
   		r²		(k² + r²)		(k² + r²)

   
   

   Correct Option: C

   Tension in thread,
   

   T =		mk²	×	gr²	=	mgk²
   		r²		(k² + r²)		(k² + r²)

   
   

---

42. The linear acceleration of the reel is

1. 1. 	gr²
      	(r² + k²)

   
   
   

   2. 	gk²
      	(r² + k²)

   
   
   

   3. 	grk
      	(r² + k²)

   
   
   

   4. 	mgr²
      	(r² + k²)

      
      

   
   
   

---

1. View Hint View Answer Discuss in Forum

   NA

   Correct Option: A

   NA

---

---

43. Instantaneous centre of a body rolling without sliding on a stationary curved surface lies.

1. 1. at the point of contact

   
   
   

   2. on the common normal at the point of contact

   
   
   

   3. on the common tangent at the point of contact

   
   
   

   4. at the centre of curvature of the stationary surface

   
   
   

---

1. View Hint View Answer Discuss in Forum

   NA

   Correct Option: A

   NA

---

44. A particle of unit mass is moving on a plane. Its trajectory, in polar coordinates, is given by r(t) = t², θ(t), where f is time. The kinetic energy of the particle at time t = 2 is

1. 1. 4

   
   
   

   2. 12

   
   
   

   3. 16

   
   
   

   4. 24

   
   
   

---

1. View Hint View Answer Discuss in Forum

   r = t²
   θ = t
   x = r cos θ = t² cost
   y = r sin θ = t² sint
   

   u =	dx	= 2t cost - t² sint
   	dt

   
   at t = 2
   u = 4 cos 2 – 4 sin 2 = – 5.301
   

   v =	dy	= 2t sin t + t² cos t
   	dt

   
   at t = 2
   v = 4 sin 2 + 4 cos 2 = 1.972
   V = √u² + v² = 5.656
   

   K.E. =	1	= mv²
   	2

   
   

   =	1	= × 1 × 5.656² = 15.99
   	2

   
   

   Correct Option: C

   r = t²
   θ = t
   x = r cos θ = t² cost
   y = r sin θ = t² sint
   

   u =	dx	= 2t cost - t² sint
   	dt

   
   at t = 2
   u = 4 cos 2 – 4 sin 2 = – 5.301
   

   v =	dy	= 2t sin t + t² cos t
   	dt

   
   at t = 2
   v = 4 sin 2 + 4 cos 2 = 1.972
   V = √u² + v² = 5.656
   

   K.E. =	1	= mv²
   	2

   
   

   =	1	= × 1 × 5.656² = 15.99
   	2

   
   

---

---

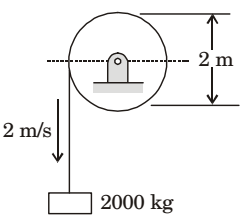
45. A mass of 2000 kg is currentiy being lowered at a velocity of 2 m/s from the drum as shown in the figure. The mass moment of inertia of the drum is 150 kg-m². On applying the brake, the mass is brought to rest in a distance of 0.5 m. The energy absorbed by the brake (in kJ) is ________.
    
    

1. 1. 14.11 kJ

   
   
   

   2. 13.11 kJ

   
   
   

   3. 12.11 kJ

   
   
   

   4. 15.11 kJ

   
   
   

---

1. View Hint View Answer Discuss in Forum

   
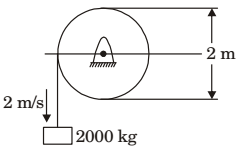
   V = rω
   

   ωi =		Vu	=	2	= 2 rad/sec
   		r		1

   
   

   Loss in KE of drum =	1	J(ω²i - ω²f)
   	2

   
   

   =	1	150(2² - 0²)
   	2

   
   = 300 Joule
   

   Loss in KE of block =	1	m(ω²i - ω²f)
   	2

   
   

   =	1	2000(2² - 0²)
   	2

   
   = 4000 Joule
   Loss in potential energy of block = mgh
   = 2000 × 9.81 × 0.5 = 9810 Joul
   Total loss of energy = 300 + 4000 + 9810
   = 14110 J
   = 14.11 kJ

   Correct Option: A

   
   V = rω
   

   ωi =		Vu	=	2	= 2 rad/sec
   		r		1

   
   

   Loss in KE of drum =	1	J(ω²i - ω²f)
   	2

   
   

   =	1	150(2² - 0²)
   	2

   
   = 300 Joule
   

   Loss in KE of block =	1	m(ω²i - ω²f)
   	2

   
   

   =	1	2000(2² - 0²)
   	2

   
   = 4000 Joule
   Loss in potential energy of block = mgh
   = 2000 × 9.81 × 0.5 = 9810 Joul
   Total loss of energy = 300 + 4000 + 9810
   = 14110 J
   = 14.11 kJ

---