26. For the same material and the mass, which of the following configurations of flywheel will have maximum mass moment of inertia about the axis of rotation OO' passing through the center of gravity.

1. 1. 

   
   
   

   2. 

   
   
   

   3. 

   
   
   

   4. 

   
   
   

---

1. View Hint View Answer Discuss in Forum

   Rim med wheel has maximum mass located away from the axis of rotation. Thus will have maximum moment of inertia.

   Correct Option: B

   Rim med wheel has maximum mass located away from the axis of rotation. Thus will have maximum moment of inertia.

---

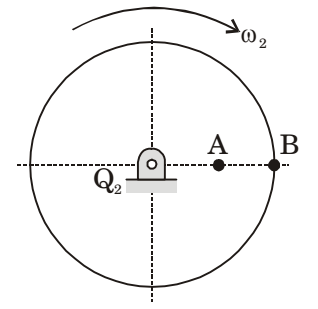
27. Figure shows a wheel rotating about Q₂. Two points A and B located along the radius of wheel have speeds of 80 m/s and 140 m/s respectively. The distance between the points A and B is 300 mm. The diameter of the wheel (in mm) is ______.
    

1. 1. 1400

   
   
   

   2. 1500

   
   
   

   3. 1600

   
   
   

   4. 1700

   
   
   

---

1. View Hint View Answer Discuss in Forum

   
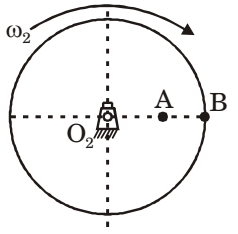
   V_A = 80 m/s, V_B = 140 m/s
   r_B – r_A = 300 ...(i)
   ω × r_A = 80
   ω × r_B = 140
   

   ∴	rB	= 1.75 ...................(ii)
   	rA

   
   Solving (i) and (ii), r_B = 700 mm.
   ∴ Diameter of wheel is 1400 mm.

   Correct Option: A

   
   V_A = 80 m/s, V_B = 140 m/s
   r_B – r_A = 300 ...(i)
   ω × r_A = 80
   ω × r_B = 140
   

   ∴	rB	= 1.75 ...................(ii)
   	rA

   
   Solving (i) and (ii), r_B = 700 mm.
   ∴ Diameter of wheel is 1400 mm.

---

---

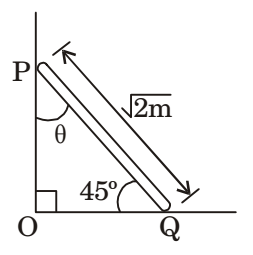
28. The rod PQ of length L = 2 m and uniformly distributed mass of M = 10 kg, is released from rest at the position shown in the figure. The ends slide along the friction less faces OP and OQ. Assume acceleration due to gravity, g = 10 m/s². The mass moment of inertia of the rod about its centre of mass and an axis perpendicular to the plane of the figure is (ML²/12). At this instant, the magnitude of angular acceleration (in radian/s²) of the rod is ______.'
    

1. 1. 7.5

   
   
   

   2. 7.8

   
   
   

   3. 7.7

   
   
   

   4. 7.6

   
   
   

---

1. View Hint View Answer Discuss in Forum

   
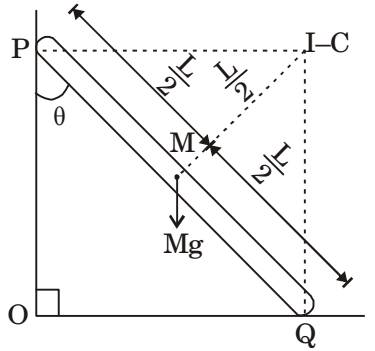
   T_I-C = T_I-C
   

   ⇒ Mgx	L	cos45° =		ICM +	ML2		α
   	2				4

   
   

   ⇒ Mg	L	cos45° =		ML2	+	ML2		α
   	2			12		4

   
   

   α =	3g	cos45°
   	2L

   
   

   α =	30	+	1		∴ g = 10	m
   	2 × V2		V2			s2

   
   

   α = 7.5	rad
   	s2

   Correct Option: A

   
   T_I-C = T_I-C
   

   ⇒ Mgx	L	cos45° =		ICM +	ML2		α
   	2				4

   
   

   ⇒ Mg	L	cos45° =		ML2	+	ML2		α
   	2			12		4

   
   

   α =	3g	cos45°
   	2L

   
   

   α =	30	+	1		∴ g = 10	m
   	2 × V2		V2			s2

   
   

   α = 7.5	rad
   	s2

---

29. The position vector OP of point P(20, 10) is rotated anti-clockwise in X-Y plane by an angle θ = 30° a such that point P occupies position Q, as shown in the figure. The coordinates (x, y) of Q are
    

1. 1. (12.32, 18.66)

   
   
   

   2. (18.66, 12.32)

   
   
   

   3. (22.32 ,8.26)

   
   
   

   4. (13.40, 22.32)

   
   
   

---

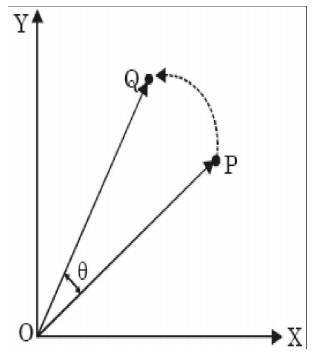
1. View Hint View Answer Discuss in Forum

   Let Co-ordinates of Q are (x', y') and rotation about x-y plane anti clockwise.
   

   X'

   =

   cosθ

   -sinθ

   X

   Y'

   sinθ

   cosθ

   Y

   
   

   X'

   =

   cos30

   -sin30

   20

   Y'

   sin30

   cos30

   10

   
   

   =		0.866	-0.5				20
   		0.5	0.866				10

   
   

   X'

   =

   0.866 × 20

   -0.5 × 10

   =

   12.32

   Y'

   0.5 × 20

   0.866 × 10

   18.66

   
   (x', y') = (12.32, 18.66)

   Correct Option: A

   Let Co-ordinates of Q are (x', y') and rotation about x-y plane anti clockwise.
   

   X'

   =

   cosθ

   -sinθ

   X

   Y'

   sinθ

   cosθ

   Y

   
   

   X'

   =

   cos30

   -sin30

   20

   Y'

   sin30

   cos30

   10

   
   

   =		0.866	-0.5				20
   		0.5	0.866				10

   
   

   X'

   =

   0.866 × 20

   -0.5 × 10

   =

   12.32

   Y'

   0.5 × 20

   0.866 × 10

   18.66

   
   (x', y') = (12.32, 18.66)

---

---

30. A wheel of mass m and radius r is in accelerated rolling motion without slip under a steady axle torque T. If the coefficient of kinetic friction is μ, the friction force from the ground on the wheel is

1. 1. μ mg

   
   
   

   2. T/r

   
   
   

   3. zero

   
   
   

   4. None of these

   
   
   

---

1. View Hint View Answer Discuss in Forum

   NA

   Correct Option: D

   NA

---