Dual Nature of Radiation and Matter
- A 200 W sodium street lamp emits yellow light of wavelength 0.6 µm. Assuming it to be 25% efficient in converting electrical energy to light, the number of photons of yellow light it emits per second is
-
View Hint View Answer Discuss in Forum
Give that, only 25% of 200 W converter electrical energy into light of yellow colour

hc 
× N = 200 × 25 λ 100
Where N is the No. of photons emitted per second, h = plank’s constant, c, speed of light.N = 200 × 25 × λ 100 hc = 200 × 25 × 0.6 × 10-6 1.5 × 1020 100 × 6.2 × 10-34 × 3 × 108 Correct Option: A
Give that, only 25% of 200 W converter electrical energy into light of yellow colour

hc 
× N = 200 × 25 λ 100
Where N is the No. of photons emitted per second, h = plank’s constant, c, speed of light.N = 200 × 25 × λ 100 hc = 200 × 25 × 0.6 × 10-6 1.5 × 1020 100 × 6.2 × 10-34 × 3 × 108
- A source of light is placed at a distance of 50 cm from a photocell and the stopping potential is found to be V0. If the distance between the light source and photocell is made 25 cm, the new stopping potential will be
-
View Hint View Answer Discuss in Forum
Since, stopping potential is independent of distance hence new stopping potential will remain unchanged i.e., new stopping potential = V0.
Correct Option: C
Since, stopping potential is independent of distance hence new stopping potential will remain unchanged i.e., new stopping potential = V0.
- For photoelectric emission from certain metal the cut-off frequency is ν. If radiation of frequency 2ν impinges on the metal plate, the maximum possible velocity of the emitted electron will be (m is the electron mass)
-
View Hint View Answer Discuss in Forum
From photo-electric equation,
hν' = hν +
Kmax ...(i)h.2v = hv + 1 mV2max 2
[∴ v' = 2v]⇒ hv = 1 mV2max 2 ⇒ vmax = 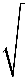
2hv m Correct Option: B
From photo-electric equation,
hν' = hν +
Kmax ...(i)h.2v = hv + 1 mV2max 2
[∴ v' = 2v]⇒ hv = 1 mV2max 2 ⇒ vmax = 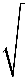
2hv m
- When the energy of the incident radiation is incredased by 20%, the kinetic energy of the photoelectrons emitted from a metal surface increased from 0.5 eV to 0.8 eV. The work function of the metal is :
-
View Hint View Answer Discuss in Forum
According to Einstein’s photoelectric equation, hν = φ0 + Kmax
We have
hν = φ0 + 0.5 ...(i)
and1.2hν = φ0 + 0.8 ...(ii)
Therefore, from above two equations φ0 = 1.0 eV.Correct Option: B
According to Einstein’s photoelectric equation, hν = φ0 + Kmax
We have
hν = φ0 + 0.5 ...(i)
and1.2hν = φ0 + 0.8 ...(ii)
Therefore, from above two equations φ0 = 1.0 eV.
- A photoelectric surface is illuminated successively by monochromatic light of wavelength λ and λ/2. If the maximum kinetic energy of the emitted photoelectrons in the second case is 3 times that in the first case, the work function of the surface of the material is :
(h = Planck's constant, c = speed of light)
-
View Hint View Answer Discuss in Forum
Photoelectric equations
Ek1max = hc - φ ....(i) λ and Ek2max = hc - φ λ/2 Ek2max = 2hc - φ ....(ii) λ
From question, Ek2max = 3Ek1max Multiplying equation (i) by 3 3Ek1max = 
hc - φ 
....(iii) λ
From equation (ii) and (iii)3hc - 3φ = 2hc - φ λ λ ∴ φ (work function) = hc 2λ Correct Option: D
Photoelectric equations
Ek1max = hc - φ ....(i) λ and Ek2max = hc - φ λ/2 Ek2max = 2hc - φ ....(ii) λ
From question, Ek2max = 3Ek1max Multiplying equation (i) by 3 3Ek1max = 
hc - φ 
....(iii) λ
From equation (ii) and (iii)3hc - 3φ = 2hc - φ λ λ ∴ φ (work function) = hc 2λ

