1. For a single server with Poisson arrival and exponential service time, the arrival rate is 12 per hour. Which one of the following service rates will provide a steady state finite queue length?

1. 1. 6 per hour

   
   
   

   2. 10 per hour

   
   
   

   3. 12 per hour

   
   
   

   4. 24 per hour

   
   
   

---

1. View Hint View Answer Discuss in Forum

   For steady state, μ > λ
   as λ = 12 customers/hour, we need to select option (d)

   Correct Option: D

   For steady state, μ > λ
   as λ = 12 customers/hour, we need to select option (d)

---

2. In a single-channel queuing model, the customer arrival rate is 12 per hour and the serving rate is 24 per hour. The expected time that a customer is in queue is minutes.

1. 1. 2.1min

   
   
   

   2. 2.5 min

   
   
   

   3. 3.5 min

   
   
   

   4. 2 min

   
   
   

---

1. View Hint View Answer Discuss in Forum

   λ = 12 /hr, μ = 24 /hr
   

   ρ =	λ	=	12	= 0.5
   	μ		24

   
   Expected time that the customer in the queue
   

   Wq =	Lq	=	Ls - ρ
   	λ		λ

   
   

   =	ρ	- ρ	=	0.5	- 0.5
   	1 - ρ			1 - 0.5
   	λ			12

   
   

   =	0.5	hr =	0.5	× 60 min
   	12		12

   
   = 2.5 min

   Correct Option: B

   λ = 12 /hr, μ = 24 /hr
   

   ρ =	λ	=	12	= 0.5
   	μ		24

   
   Expected time that the customer in the queue
   

   Wq =	Lq	=	Ls - ρ
   	λ		λ

   
   

   =	ρ	- ρ	=	0.5	- 0.5
   	1 - ρ			1 - 0.5
   	λ			12

   
   

   =	0.5	hr =	0.5	× 60 min
   	12		12

   
   = 2.5 min

---

---

3. Which one of the following is NOT a decision taken during the aggregate production planning stage?

1. 1. Scheduling of machines

   
   
   

   2. Amount of labour to be committed

   
   
   

   3. Rate at which production should happen

   
   
   

   4. Inventory to be carried forward

   
   
   

---

1. View Hint View Answer Discuss in Forum

   NA

   Correct Option: B

   NA

---

4. The Product structure of an assembly P is shown in the figure.
   
   Estimated demand for end product P is as follows: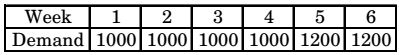
   
   Ignore lead times for assembly and subassembly. Production capacity (per week) for component R is the bottleneck operation. Starting with zero inventory, the smallest capacity that will ensure a feasible production plan up to week 6 is

1. 1. 1000

   
   
   

   2. 1200

   
   
   

   3. 2200

   
   
   

   4. 2400

   
   
   

---

1. View Hint View Answer Discuss in Forum

   The M.R.P. for R is given by
   
   If 6P – 12800 ≥ 0 then the capacity is feasible
   ∴ Minimization, 6P = 12800
   ⇒ P ≅ 2200

   Correct Option: C

   The M.R.P. for R is given by
   
   If 6P – 12800 ≥ 0 then the capacity is feasible
   ∴ Minimization, 6P = 12800
   ⇒ P ≅ 2200

---

---

5. Following data refers to the activities of a project, where, node 1 refers to the start and node 5 refers to the end of the project
   
   The critical path (CP) in the network is

1. 1. 1-2-3-5

   
   
   

   2. 1-4-3-5

   
   
   

   3. 1-2-3-4-5

   
   
   

   4. 1-4-5

   
   
   

---

1. View Hint View Answer Discuss in Forum

   
   Critical path ⇒ 1 – 4 – 3 – 5
   Time takes = 8 days

   Correct Option: B

   
   Critical path ⇒ 1 – 4 – 3 – 5
   Time takes = 8 days

---