Direction: 16 teams have been invited to participate in the ABC Gold Cup cricket tournament. The tournament is conducted in 2 stages. In the first stage, the teams are divided into two groups. Each group consists of 8 teams, with each team playing every other team in its group exactly once. At the end of the first stage, the top 4 teams from each group advance to the 2nd stage while the rest are eliminated. The second stage comprises of several rounds. A round involves one match for each team. The winner of a match in a round advances to the next round, while the loser is eliminated. The team that remains undefeated in the second stage is declared the winner and claims the Gold Cup. The tournament rules are such that each match results in a winner and a loser with no possibility of a tie. In the first stage a team earns 1 point for each win and no points for a loss. At the end of the first stage teams in each group are ranked on the basis of total points to determine the qualifiers advancing to the next stage. Ties are resolved by a series of complex tie-breaking rules so that exactly 4 teams from each group advance to the next stage.
-
The minimum number of wins needed for a team in the first stage to guarantee is advancement to the next stage is:
-
- 5
- 6
- 7
- 4
Correct Option: D
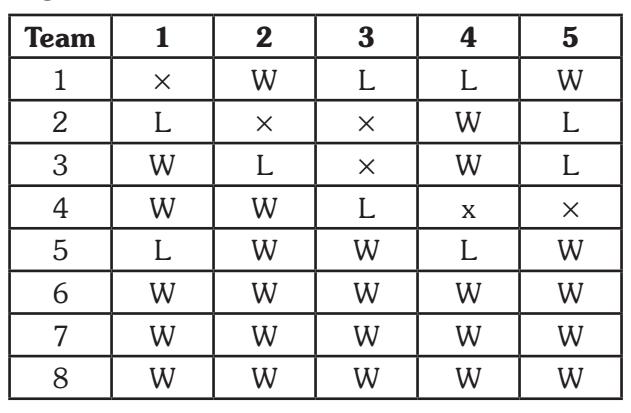
From the given information we can complete the following table it is clear that if a team wins 5 games, then also there is no guarantee of its advancement to the next stage, since only 4 teams can go to the next stage.
Note: In the table W → Wins, L → Loose, × → No match (as example there can not be match between 1& and 1, 2 and 2 and so on)
The above is one of such combination. Since after winning 5 matches too, there is no guarantee to advancement, so the answer must be 6, because no two teams can get 7 points each.