Concrete structures miscellaneous

Concrete structures miscellaneous

Easy Questions

Concrete Structures

Concrete structures miscellaneous
  1. In a reinforced concrete section, the stress at the extreme fibre in compression is 5.80 MPa. The depth of neutral axis in the section is 58 mm and the grade of concrete is M25.
    Assuming linear elastic behaviour of the concrete, the effective curvature of the section (in per mm) is








  1. View Hint View Answer Discuss in Forum

    Modules of elasticity, E = 5000 √ fck
    = 5000 √25
    = 25000 N/mm2

    M
    		=
    E
    		=
    σ
    IRy

    Curvature,
    I
    		=
    σ
    REy

    =
    5.8
    E.Xu

    =
    5 - 8
    58 × 25000

    = 4 × 10–6/mm

    Correct Option: C

    Modules of elasticity, E = 5000 √ fck
    = 5000 √25
    = 25000 N/mm2

    M
    		=
    E
    		=
    σ
    IRy

    Curvature,
    I
    		=
    σ
    REy

    =
    5.8
    E.Xu

    =
    5 - 8
    58 × 25000

    = 4 × 10–6/mm

  1. A column of size 450 mm × 600 mm has unsupported length of 3.0 m and is braced against side sway in both directions. According to IS 456: 2000, the minimum eccentricities (in mm) with respect to major and minor principal axes are:








  1. View Hint View Answer Discuss in Forum

    As per IS 456: 2000

    emin =
    L
    		+
    D
    		or 20 mm, whichever is minimum.
    50030

    exx =
    3000
    		+
    600
    		26 mm > 20 mm,
    50030

    eyy =
    300
    		+
    450
    		21 mm > 20 mm,
    50030

    Both exx and eyy are greater than 20 mm.
    ∴ we take 20 mm each.

    Correct Option: A

    As per IS 456: 2000

    emin =
    L
    		+
    D
    		or 20 mm, whichever is minimum.
    50030

    exx =
    3000
    		+
    600
    		26 mm > 20 mm,
    50030

    eyy =
    300
    		+
    450
    		21 mm > 20 mm,
    50030

    Both exx and eyy are greater than 20 mm.
    ∴ we take 20 mm each.

  1. In a pre-stressed concrete beam section shown in the figure, the net loss is 10% and the final pre-stressing force applied at X is 750 kN. The initial fiber stresses (in N/mm2 ) at the top and bottom of the beam were:








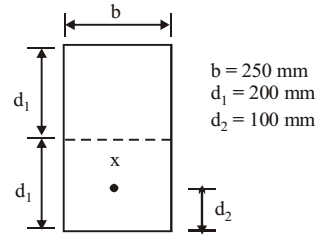
  1. View Hint View Answer Discuss in Forum

    Final force = 750 kN
    Loss = 10%
    ∴ Initial force

    =
    750
    		= 833.33 kN
    1 -
    10
    100

    Top and bottom stress
    =
    P
    		±
    m
    AZ

    =
    833.33
    		× 10-3 ±
    833.33 × 103 × 100 × 6
    250 × 400250 × 4002

    = 8.33 ± 12.5
    ∴ Top stress = 8.33 – 12.5 = –4.17 (tension)
    Bottom stress = 8.33 + 12.5 = 20.83 (compression)

    Correct Option: D

    Final force = 750 kN
    Loss = 10%
    ∴ Initial force

    =
    750
    		= 833.33 kN
    1 -
    10
    100

    Top and bottom stress
    =
    P
    		±
    m
    AZ

    =
    833.33
    		× 10-3 ±
    833.33 × 103 × 100 × 6
    250 × 400250 × 4002

    = 8.33 ± 12.5
    ∴ Top stress = 8.33 – 12.5 = –4.17 (tension)
    Bottom stress = 8.33 + 12.5 = 20.83 (compression)

Direction: At the limit state of collapse, an R.C. beam is subjected to flexural moment 200 kN-m, shear force 20 kN and torque 9 kN-m. The beam is 300 mm wide and has gross depth of 425 mm, with an effective cover of 25 mm. the equivalent nominal shear stress (τve) as calculated by using the desing code turns out to be lesser than the design shear strength (τc) of the concrete.

  1. The equivalent shear force (Ve) is








  1. View Hint View Answer Discuss in Forum

    Ve = Vu + 1.6
    Tu
    b

    = 20 +
    (1.6) × 9
    		= 68 kN
    300 × 10-3

    Correct Option: D

    Ve = Vu + 1.6
    Tu
    b

    = 20 +
    (1.6) × 9
    		= 68 kN
    300 × 10-3