-
A flat-faced follower is driven using a circular eccentric can rotating at a constant angular velocity ω. At time t = 0, the vertical position of the follower is y(0) = 0, and the system is in the configuration shown below.
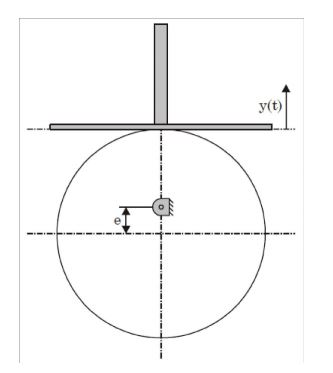
The vertical position of the follower face, y(t) is given by
-
- e sin ωt
- e(1 + cos 2ωt)
- e sin 2 ωt
- e(1 – cos ωt)
- e sin ωt
Correct Option: D
As the follower starts moving, the displacement Y can be shown as marked in the diagram
Y = QS – QT
= RU – QT (∵ QS ∥ RU)
= (PR – PU) – QT ...(i)
PR = R'
⇒ PU = e cos θ
QT = (R' – e) from geometry
Now, putting the above values in equation (i) Y = (R' – e cos θ) – (R' – e)
= e – e cos θ
= e(1 – cos θ)
⇒ Y = e(1 – cos ωt) (In time ‘t’,θ = ωt)

