Correct Option: A

The given circuit: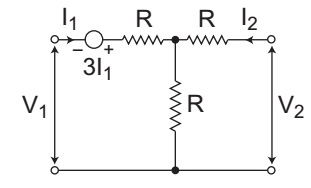

Applying KVL in the input side, we get
V₁ + 3I₁ + I₁R + (I₁ + I₂) R = 0
or
V₁ = I₁ (2R – 3) + I₂R . . .. .…(i)
Again applying KVL in the output side
V₂ = I₂R + (I₁ + I₂)R
or
V₂ = I₁R + I₂.2R …. . . . .(ii)
From equation (i) and (ii)
Z₁₁ = 2R – 3, Z₁₂ = R
Z₂₁ = R, Z₂₂ = 2R
● For the network to be symmetric
Z₁₁ = Z₂₂ (But here Z₁₁ = 2R – 3 and Z₂₂ = 2R)
So, network is not symmetric.
● For the network to be reciprocal:
Z₁₂ = Z₂₁ (Here Z₁₂ = Z₂₁ = R)
So, network is reciprocal.
Thus, we conclude that the given network is reciprocal but not symmetric.